Sensitivity of the orographic precipitation across the Australian Snowy Mountains to regional climate indices
Fahimeh Sarmadi A B E , Yi Huang C D , Steven T. Siems A B and Michael J. Manton AA School of Earth, Atmosphere and Environment, 9 Rainforest Walk, Monash University, Melbourne, Vic. 3800, Australia.
B Australian Research Council (ARC) Centre of Excellence for Climate System Science, Monash University, Melbourne, Vic., Australia.
C School of Earth Sciences, The University of Melbourne, Melbourne, Vic., Australia.
D Australian Research Council Centre of Excellence for Climate Extremes, Melbourne, Vic., Australia.
E Corresponding author. Email: fahimeh.sarmadi@monash.edu
Journal of Southern Hemisphere Earth Systems Science 69(1) 196-204 https://doi.org/10.1071/ES19014
Submitted: 1 April 2019 Accepted: 1 July 2019 Published: 11 June 2020
Journal Compilation © BoM 2019 Open Access CC BY-NC-ND
Abstract
The wintertime (May–October) precipitation across south-eastern Australia, and the Snowy Mountains, was studied for 22 years (1995–2016) to explore the sensitivity of the relationships between six established climate indices and the precipitation to the orography, both regionally and locally in high-elevation areas. The high-elevation (above 1100 m) precipitation records were provided by an independent network of rain gauges maintained by Snowy Hydro Ltd. These observations were compared with the Australian Water Availability Project (AWAP) precipitation analysis, a commonly used gridded nationwide product. As the AWAP analysis does not incorporate any high-elevation sites, it is unable to capture local orographic precipitation processes. The analysis demonstrates that the alpine precipitation over the Snowy Mountains responds differently to the indices than the AWAP precipitation. In particular, the alpine precipitation is found to be most sensitive to the position of the subtropical ridge and less sensitive to a number of other climate indices tested. This sensitivity is less evident in the AWAP representation of the high-elevation precipitation. Regionally, the analysis demonstrates that the precipitation to the east of the Snowy Mountains (the downwind precipitation) is weakly correlated with the upwind and peak precipitation. This is consistent with previous works that found that the precipitation in this downwind region commonly occurs from mechanisms other than storm systems passing over the mountains.
1 Introduction
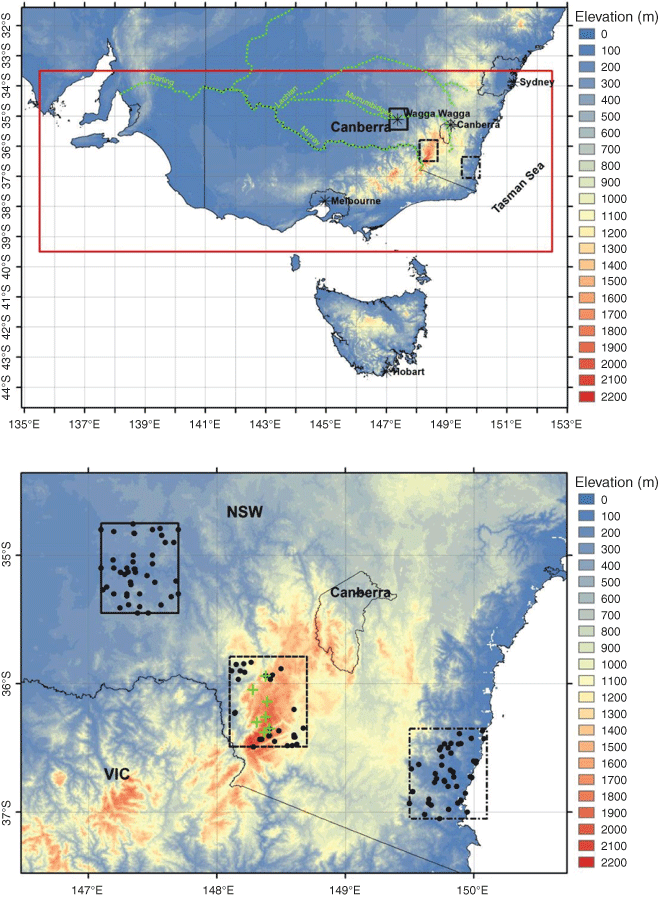
The Australian Alps are the highest part of the continental divide along the eastern seaboard, known as the Great Dividing Range, and plays a crucial role in the weather across the densely populated south-eastern seaboard. The Great Dividing Range forms the headwaters of many of the major rivers in the Murray–Darling Basin and underpins many unique natural ecosystems of the high-mountain catchments with some of the richest biodiversity areas on the mainland. The Alpine water accounts for 29% of the annual average inflow yield of the Murray–Darling Basin (Worboys and Good 2011). The Snowy Mountains, which reside along the Great Dividing Range, are the tallest mountains among the few Alpine regions in Australia (Fig. 1, top panel). Precipitation over the Snowy Mountains has been of great interest among researchers, given its central role in feeding some of the major river systems of the Murray–Darling Basin, as well as providing hydroelectric power for much of eastern Australia.
Two prolonged periods of dry conditions have been experienced in south-eastern Australia (south of 33.5°S and east of 135.5°E) in the past 100 years. An 11-year (1935–45) period and a 13-year (1997–2009) period both had rainfall deficits of above 10%, relative to the 1900–2009 long-term average. Much of south-west and south-east Australia underwent below-average to record-low rainfall during the peak of the Millennium Drought, which is defined by van Dijk et al. (2013) as the period 2001–09: the longest consecutive series of years with below-median rainfall in south-east Australia since at least 1900. The Millennium Drought had a significant impact on the Australian economy and led to large declines in agricultural employment and rural exports (Lu and Hedlry 2004). According to the Bureau of Meteorology (BOM) data (http://www.bom.gov.au/climate/drought/), in 2006, south-east Australia experienced its second-driest year on record since 1900, with below-normal annual precipitation. Nicholls (2005) reported a decreasing trend in both maximum, from ~210 to ~190 cm, and spring snow depth, from ~175 to ~100 cm, in the Snowy Mountains over a 40-year period beginning in 1962. A decline of ~10% was observed in the maximum snow depth over this period. A much larger decrease in spring snow depth (~40%) was also observed, mostly attributed to the melting of the snow due to a combination of a slight decline in winter precipitation and a strong warming trend during July–September.
Risbey et al. (2009) suggested that precipitation across Australia, and, in particular, south-east Australia, is generally governed by large-scale climate drivers such as the El Niño–Southern Oscillation (ENSO) Index, Southern Annular Mode (SAM), Indian Ocean Dipole (IOD) and the Atmospheric Blocking Index (ABI) at the longitude of 140°E. They found the ABI to be the dominant climate driver for winter precipitation across south-east Australia. Timbal and Drosdowsky (2013) linked the spatial and temporal rainfall decline in south-east Australia to the position (STR-P) and intensity (STR-I) of the subtropical ridge during 1997–2009. Grose et al. (2015) found the same relationship in the historical Coupled Model Intercomparison Project Phase 5 simulations. Several studies have documented the sensitivity of precipitation in south-east Australia to the Southern Oscillation Index (SOI, as an indicator of ENSO), indicating higher mean monthly precipitation during La Niña events (e.g. Gallant et al. 2012; Murphy and Timbal 2008; Ummenhofer et al. 2011; Cai et al. 2011; Pepler et al. 2014; Theobald and McGowan 2016).
For many of these studies, the precipitation over south-east Australia has commonly been defined from a precipitation analysis product, such as the Australian Water Availability Project (AWAP; Jones et al. 2009), for a specified domain. The correlation of the average precipitation over the domain with the specific climate index establishes the strength of any relationship. As there is no single fixed definition of south-east Australia, these studies have commonly defined a broad domain that can average over orographic and nonorographic regions, even though the Snowy Mountains have been found to greatly affect the precipitation, both regionally (e.g. Timbal 2010; Pepler et al. 2014) and locally over the peaks (Chubb et al. 2011; Huang et al. 2018). On a regional scale, mountains can block an advancing air mass and its precipitation, diverting it rather than having it pass over the top. Locally, orographic precipitation can occur from a variety of dynamic and microphysical processes that are not present at upwind and downwind sites. For instance, Houze (2012) identified 12 distinct orographic processes that can create, enhance and/or redistribute precipitation.
Detailed case studies of wintertime precipitation events over the Great Dividing Range have found that postfrontal orographic rainfall can make a substantial contribution to the total precipitation (Chubb et al. 2012; Sarmadi et al. 2019). Chubb et al. (2011) detailed that the Southern Ocean serves as the source of water for this postfrontal air mass, being converted to precipitation when lifted over the Snowy Mountains. Overall, they found that the wintertime precipitation over the Snowy Mountains was a factor of ~4, greater than for an upwind site over the Mallee. Chubb et al. (2016) employed a high-density network of rain gauges over the Snowy Mountains to evaluate the AWAP precipitation product finding that the analysis underestimated precipitation on the upwind slopes of the mountains, while slightly overestimating the downwind precipitation. Although the AWAP product makes corrections for altitude, it does not account for upwind–downwind effects. Lewis et al. (2018) studied these biases over western Tasmania demonstrating that the AWAP precipitation analysis may not fully capture the common effect of orographic blocking. Huang et al. (2018) evaluated the BOM operational forecasts of precipitation with the same network of high-elevation rain gauges over the Snowy Mountains, finding that the unique microphysics over the Snowy Mountains (Morrison et al. 2013) may be important in the generation of precipitation in the Alpine regions. Sarmadi et al. (2019) studied the sensitivity of numerical simulations of precipitation over the Snowy Mountains to the microphysics scheme, highlighting how the conversion of commonly observed supercooled liquid water to ice affected the distribution of precipitation across the mountains.
The complexity of wintertime orographic precipitation over the Snowy Mountains suggests that it may not be well represented by a precipitation analysis that does not directly incorporate high-elevation surface observations, such as AWAP (Chubb et al. 2016). Accordingly, the correlation between the average regional precipitation and a given climate index may not apply to the high-elevation orographic precipitation. Similarly, a single correlation over a broad region may not reveal variations arising from the orography. Given the importance of the precipitation across south-east Australia, and the Snowy Mountains in particular, the aim of this study is to explore the sensitivity of the relationships between climate indices and the precipitation to the orography, both regionally and locally at high-elevation areas. Unlike the previous climate studies mentioned, independent high-elevation rain gauge observations are employed in this analysis.
2 Precipitation data sources
The analysis is limited to the 22-year (1995–2016) period, wintertime only (May–October) being constrained by the availability of high quality, high elevation, surface observations from Snowy Hydro Ltd. (SHL).
2.1 Snowy Hydro Ltd. ground-based wintertime (May–October) precipitation data set
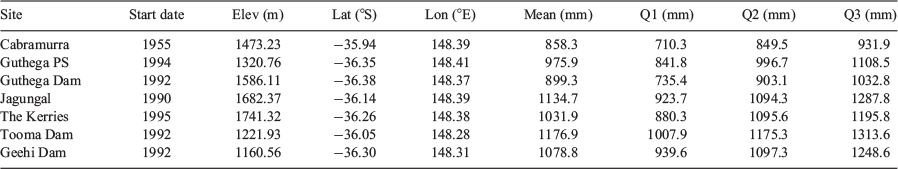
Local, high-elevation precipitation across the Snowy Mountains is obtained from seven well-maintained weather stations above 1100 m, operated by SHL. Half-hourly precipitation amounts are accumulated from 0900 hours local time (2300 UTC) to the same time of the next day, and then these daily precipitation values are aggregated to yield monthly totals. For the 22-year winter period (May–October) considered in this study, ~80% of all days have at least five stations that had a suitable quality flag contributing to the mean value; data flagged as unsuitable are removed. No systematic bias in missing or flagged data has previously been noted. The locations, elevations, operating year of the gauges and their fairly uniform long-term mean wintertime precipitation are shown in Table 1; Figure 1 shows a map of their locations. A more complete description of the instrumentation and this data set can be found in Chubb et al. (2016). These seven high-elevation surface records have commonly been averaged together to represent the precipitation across the Snowy Mountains (e.g. Sarmadi et al. 2019) and is hereafter referred to as SHL 7-site average. The precipitation from these sites is not employed in the production of the AWAP analysis, making it an independent data set that can be employed for evaluation purposes (Chubb et al. 2016).
|
2.2 The Australian Water Availability Project
The AWAP (Jones et al. 2009) provides a national gridded product with the aim of monitoring the terrestrial water balance across the entire Australian continent. The AWAP analysis interpolates the Australian BOM rainfall gauge data with splines at a spatial resolution of 0.05° × 0.05° from 1900 to the present. The average precipitation of the seven AWAP grid cells closest to the seven Snowy Hydro high-elevation rain gauges (AWAP 7-site average) is used to study the sensitivity to local orographic processes. Following Chubb et al. (2011), a high-elevation domain (Peak-domain) is defined (35.4–36.8°S and 147.8–149°E) over the Snowy Mountains, which will also be used to study the sensitivity to local orographic processes.
The Peak-domain is also used to study the sensitivity of the regional precipitation to orographic processes along with an upwind domain (West-domain: 34.4–35.8°S and 146.8–148°E) and a downwind domain (East-domain: 36.0–37.4°S and 149.2–150.4°E). The West-domain and East-domain are close to the Snowy Mountains but are at low elevations. Finally, a broad, regional domain covering SE Australia (33.5–39.5°S and 135.5–152.5°E) is defined (SEA-domain), which incorporates both orographic and nonorographic terrains. The domains defined in this study are shown in Fig. 1.
2.3 High-elevation precipitation
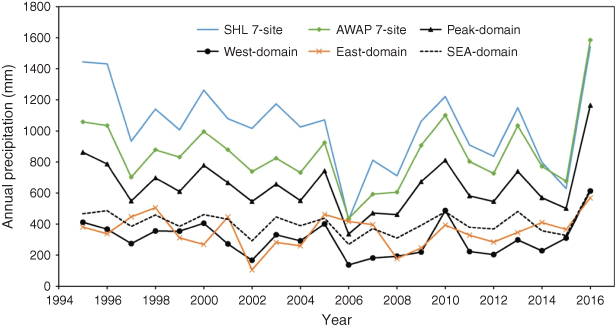
Figure 2 shows the wintertime precipitation over the 22-year period for all four domains and two site averages (SHL 7-site average and AWAP 7-site average). Wintertime precipitation over the high elevations of the Snowy Mountains (SHL 7-site average) is highly variable with extremes of 430 mm in 2006 and 1540 mm in 2016 occurring within the 22-year period.
|
Three high-elevation precipitation areas (SHL 7-site average, AWAP 7-site average and Peak-domain) have the greatest precipitation for each of the 22 years, as expected, again demonstrating the importance of the orographic enhancement. Given that the Peak-domain is averaged over the entire box, it includes terrain below 1100 m elevation. Accordingly, it has less precipitation than the other two high-elevation averages. The correlation between the AWAP 7-site and the Peak-domain is 0.99; however, both AWAP 7-site average and Peak-domain are essentially using the same lower elevation sites. Differences between these two domains largely reflect the altitude scaling within the AWAP algorithm (Chubb et al. 2016), suggesting that they will display similar relationships to any climate indices. Ideally, the SHL 7-site average and AWAP 7-site average should be quite similar. In practice, however, the SHL 7-site average precipitation is greater for 20 of the 22 years, further illustrating the shortcomings of the AWAP analysis to capture local orographic enhancement when it is not directly observed (Chubb et al. 2016). Despite this shortcoming, the SHL 7-site average is strongly correlated (0.85) with both the AWAP 7-site average and the Peak-domain.
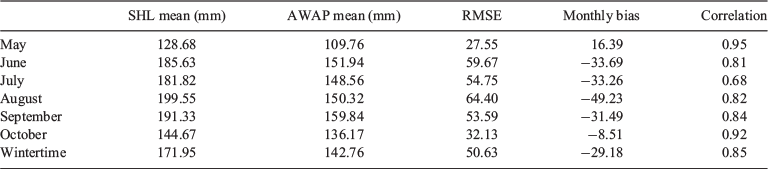
The monthly progression of the SHL 7-site and AWAP 7-site average precipitation may be further explored to better illustrate their differences (Table 2). The wintertime precipitation follows a seasonal cycle similar to that in Chubb et al. (2011) with the peak precipitation in August (199.55 mm for SHL 7-site and 150.32 mm for AWAP 7-site). For the 6-month average, the AWAP 7-site (142.76 mm) is ~17% less than SHL 7-site (171.95 mm). The overall root mean square error (RMSE) is 50.6 mm with a bias of −29.2 mm. The RMSE and bias values similarly follow a seasonal cycle, peaking in midwinter. We note that the SHL observations are not incorporated into the AWAP analysis (Chubb et al. 2016). Further, the BOM rain gauges used in AWAP are unable to record frozen precipitation rates greater than ~3 mm/h (Gorman 2003). Lower interquartile range (IQR; 25th–75th percentile) and standard deviation values in the AWAP 7-site average (IQR = 79 mm, SD = 63 mm) in comparison with the SHL 7-site average (IQR = 115, SD = 78 mm) reflect weaker variability in the AWAP product. It is expected that site measurements (SHL 7-site average) will display greater variability than gridded analysis products (AWAP 7-site average) in complex terrain (Chubb et al. 2016).
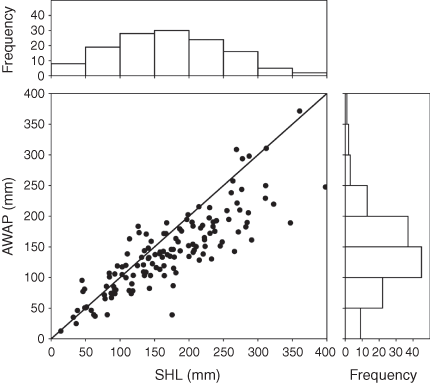
Figure 3 shows the marginal distributions of the monthly precipitation from SHL 7-site average and AWAP 7-site average, which are displayed on the horizontal and vertical axis of a scatterplot respectively. The identity line is added for reference, showing an underestimation by AWAP of up to 77% for wintertime monthly precipitation over seven gauges. This underestimation by AWAP occurs 75% of the time, leading to an underestimation of 24% on average.
|
2.4 Regional precipitation
Looking at the regional effect of orography on precipitation, the East-domain, West-domain and SEA-domain all have less wintertime precipitation than the high-elevation areas, as expected (Fig. 2). Over the 22-year period, the mean annual rainfall of SHL 7-site average is ~3 times greater than the West-domain. Table 3 shows that the West-domain correlates relatively strongly with the Peak-domain (0.75) and the SHL 7-site average (0.62), as anticipated. The weaker correlation with the SHL 7-site average further illustrates that any local orographic enhancement to precipitation may not be captured in the AWAP analysis.
On average, the East-domain is wetter than the West-domain, which may be counterintuitive. Although downstream precipitation is commonly expected to be drier than an upwind site, as water is removed in transit, the opposite can also occur depending on the exact mechanisms that are driving the precipitation (Houze 2012). This suggests that the wintertime precipitation in the East-domain is likely to be arising from different events or systems than over the West-domain. The East-domain is poorly correlated with all of the others, particularly the SHL 7-site average at only 0.01 correlation, which further suggests that the wintertime precipitation events within this domain are not related to those in the upwind and high-elevation domains. These findings are in alignment with Timbal (2010), who showed that the eastern seaboard area (coastal strip on the eastern side of the Great Dividing Range, covering the East-domain in this study) does not appear to have the same strong relationship with the key climate indices as in other regions of south-east Australia. For instance, the intensity of the subtropical ridge does not significantly impact the rainfall in this area (dissimilar to other parts of south-east Australia), whereas a southward shift of the subtropical ridge likely causes rainfall to increase in the region.
The broader, regional SEA-domain is more strongly correlated with the West-domain (0.74) and the Peak-domain (0.74) than the East-domain (0.44), which is expected; the SEA-domain largely covers the terrain to the west of the Great Dividing Range.
3 Climate indices
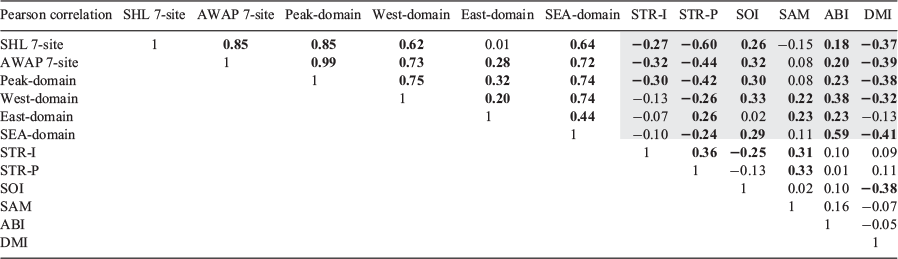
In this study, we consider the four climate indices discussed in Risbey et al. (2009), namely the SOI, the SAM, the ABI and the IOD. Further, we consider the intensity (STR-I) and location (STR-P) of the subtropical ridge, as defined in Timbal and Drosdowsky (2013). Given that the aim of this research is to explore the sensitivity of orographic precipitation to well-known climate indices, it is worthwhile to first examine the cross-correlation coefficients between these six large-scale indices (Table 3, bottom six rows). Statistically significant correlations at P = 0.05 are in bold. Observed interactions between climate indices may confound the interpretation of correlations between the climate indices and the precipitation.
The cross-correlation coefficients for the six winter months over the 22-year period suggest a large degree of independence between all indices with the greatest correlation (−0.38) found between the IOD and SOI. The IOD (also known as the Dipole Mode Index or DMI) is a measure of the anomalous zonal sea surface temperature gradient across the equatorial Indian Ocean and has commonly been linked with the SOI, a measure of pressure difference anomaly between Tahiti and Darwin (e.g. Ashok et al. 2003).
The positive cross-correlation between the STR-I and STR-P (0.36) is not unexpected either; Timbal and Drosdowsky (2013) found a significant correlation between the two STR series from April to December (1890–2009). Cai et al. (2011) found that a positive IOD tended to increase both the STR-I and STR-P, which is also found over this limited time period (cross-correlations of 0.09 and 0.11 respectively), but not statistically significant.
The ABI is another regional synoptic feature of the winter circulation. It depends on the zonal components of the mean 500-hPa wind at several latitudes. The mathematical expression of this index is

where UL represents the zonal component of the mean 500-hPa wind at latitude L (Pook and Gibson 1999). Following Risbey et al. (2009), wind data from the National Centers for Environmental Prediction–National Center for Atmospheric Research (NCEP–NCAR reanalysis data set) are utilized at 140°E (a typical longitude for Atmospheric Blocking in the Australian region) to compute the ABI. Over the 22-year period, the ABI is not found to be significantly correlated with any of the other climate indices.
The last relationship of significance between climate indices is between the SAM and the STR-I (0.31) and STR-P (0.33), which is also understandable. The SAM reflects the poleward contraction of the westerly belt that encircles Antarctica and is found to be a dominant mode of atmospheric variability in the mid/high latitudes in the Southern Hemisphere (Thompson and Solomon 2002). Marshall (2003) calculated this index using the mean sea level pressure observations from six stations as a proxy of the zonal mean at both 40 and 65°S from 1958 to present, and their calculated output is used here. The latitude of the westerly belt over the Southern Ocean is not independent of the latitude or strength of the subtropical ridge (CSIRO and Bureau of Meteorology 2015).
4 Impact of climate indices on precipitation
4.1 High-elevation precipitation
The shaded region of Table 3 records the correlations between monthly wintertime precipitation (SHL 7-site average, AWAP 7-site average and the four domains) and the six climate indices. Focussing on the three high-elevation domains, the AWAP 7-site average and Peak-domain behave similarly against all six climate indices, as expected. The peak correlation is between the STR-P and AWAP 7-site average (−0.44), which is understandable. More southerly subtropical ridge may suppress convection that is needed for heavier precipitation. This suppression is even stronger for the SHL 7-site average (−0.60); local orographic enhancement at the high elevations over the Snowy Mountains may be particularly sensitive to suppression from the subtropical ridge. The strength of the subtropical ridge (STR-I) is also significantly correlated with these high-elevation sites at −0.27, −0.32 and −0.30 for the SHL 7-site average, AWAP 7-site average and the Peak-domain respectively.
The May–October cycle of the STR-P and STR-I averaged across the periods of this study (1995–2016) has been plotted and compared with the long-term (1890–2015) climatology of Timbal and Drosdowsky (2013). The trend of monthly mean subtropical ridge over the 22-year period displays a noticeable anomaly towards greater intensity and more southerly location (not shown). A similar finding was also reported by Timbal and Drosdowsky (2013) across a period of low rainfall (1997–2009) associated with the Millennium Drought, with a noticeable anomaly in autumn to early winter. This shift in the location of the STR-P has been attributed to the poleward expansion of the descending arm of the southern hemisphere Hadley cell (e.g. Seidel et al. 2008; Nguyen et al. 2013).
Beyond the STR-P, the next strongest climate driver of significance is the IOD at −0.37, −0.39 and −0.38 for the SHL 7-site average, AWAP 7-site average and Peak-domain respectively. The IOD, the sea surface temperature anomaly difference between the tropical western and south-eastern Indian Ocean, has commonly been found to modulate rainfall across south-eastern Australia (e.g. Ashok et al. 2003; Ummenhofer et al. 2011; Pepler et al. 2014).
Measuring the anomaly of pressure difference between Tahiti and Darwin is related to the location of deep convection over the tropical Pacific Ocean and the Walker circulation. The ensuing circulation has been found to change weather patterns across the globe through a variety of proposed teleconnections. The SOI is a strong climate driver of precipitation across much of Queensland (e.g. Risbey et al. 2009). The SOI has a significant positive correlation with rainfall over southern Australia during the late winter and early spring (Risbey et al. 2009). Negative SOI values (El Niño conditions) generally decrease the mean rainfall in southern Australia in the winter and spring months (Murphy and Timbal 2008; Nicholls 2010). The correlation between the SOI and high-elevation precipitation is significant at 0.26, 0.32 and 0.30 for the SHL 7-site average, AWAP 7-site average and the Peak-domain respectively.
Atmospheric Blocking at 140°E is often linked to extended dry periods in southern Australia during winters (Pook et al. 2006). For the 22-year period examined in this study, relatively weak, but significant correlations were found between the precipitation over the high elevation and the ABI.
The SAM has previously been linked to winter rainfall over southern Australia (e.g. Meneghini et al. 2007). Chubb et al. (2011) reported ~30% less wintertime (May–September 1990–2009) precipitation during the positive phase of SAM in the high-elevation gauges over the Snowy Mountains (SHL 7-site average). The positive phase of SAM shifts the belt of strong westerly winds poleward, whereby diminishing westerly winds over south-east Australia during winter (Hendon et al. 2007). Conversely, a negative SAM brings more fronts and moisture to the southern portion of Australia. This relationship, however, is not seen to extend to the high-elevation domains at a level of statistical significance for the period of this analysis.
4.2 Regional precipitation
Finally, we turn our attention to the correlation between the climate indices and the regional precipitation domains (West-domain, East-domain and SEA-domain). Once again, the East-domain behaves quite differently from the other domains (including the high-elevation areas) for many of the climate indices, largely consistent with Timbal et al. (2010). The East-domain is positively correlated with the STR-P and is not significantly correlated against either the SOI or the IOD.
The West-domain and the SEA-domain largely have similar behaviour to one another, except against the SAM index. The West-domain and SEA-domain do behave differently from the high-elevation precipitation areas for STR-I (no significance), STR-P (weaker correlation), SAM (stronger correlation) and ABI (stronger correlation). It is worth highlighting the strong positive correlation between SEA-domain and the ABI at 0.59, which is much higher than the correlation of the ABI with any of the other three domains and two 7-site averages. This strong relationship is consistent with Risbey et al. (2009).
5 Conclusions
The primary aim of this research has been to examine the relationship between the six established climate indices on precipitation across south-east Australia with a particular focus on the orographic precipitation over the Snowy Mountains. The analysis explores not only the precipitation generated at the high-elevation alpine sites, as observed by the SHL rain gauges, but also the changes in the regional precipitation when moving across the Snowy Mountains. Three high-elevation precipitation areas (SHL 7-site average, AWAP 7-site average and Peak-domain) are considered, as well as an upwind area (West-domain), a downwind area (East-domain) and a single, broad regional domain that covers all of the SEA-domain, including all orographic regions. The SHL 7-site average is produced from independent surface observations made by SHL. These data are available for a 22-year period (1995–2016), wet season months only (May–October). Precipitation for the AWAP 7-site average and the other four domains is taken from the AWAP product. Although a 22-year period is admittedly short for undertaking climate analysis, the behaviour of the precipitation and the climate indices over this 22-year period is largely consistent with studies covering longer periods of time, where orographic precipitation was not explicitly considered.
Looking first at the regional effect of the Great Dividing Range on the impact of the various climate indices, the downwind precipitation (East-domain) is not correlated with the upwind (West-domain) or high-elevation (Peak-domain) precipitation. This suggests that the precipitation over the East-domain occurs from different weather systems from those that are commonly observed to move from the west across south-east Australia during the wet season (Timbal 2010). Correspondingly, the climate indices typically have vastly different relationships with this precipitation than with the upwind and high-elevation domains. For example, there is a positive correlation with the STR-P for this domain. The STR-P is negatively correlated with the others. In addition, the East-domain is not significantly correlated with either the SOI or the IOD; this contrasts with the observed strong significant relationships between these two indices and the other areas. This is likely due to the fact that the IOD tends to influence Australian rainfall via the sea surface temperature anomalies, setting up in the region northwest of Australia (Risbey et al. 2009).
It may also be concluded that there is limited value in including this portion of south-east Australia in a large regional domain (SEA-domain) as is commonly done. Mixing regions of different behaviours greatly complicates a deeper understanding of observed relationships.
Looking at the difference in correlations between the upwind (West-domain) and broad (SEA-domain) and the high elevation areas (SHL 7-site, AWAP 7-site and Peak-domain), some immediate differences are evident. The high-elevation sites are more sensitive to the STR-P, STR-I and SAM but less sensitive to the SOI and ABI.
Focussing exclusively on the high-elevation areas, the SHL 7-site average incorporates only the high-elevation (above 1100 m) surface sites from Snowy Hydro. The two AWAP-based precipitation products (AWAP 7-site average and Peak-domain) do not have access to these observations, ingesting observations from lower elevations into the product. These two AWAP-based precipitation products behave similarly across all aspects of this study. Most notably, the SHL 7-site average is most strongly correlated with the STR-P (0.60) and more weakly correlated with the STR-I, SOI and ABI than the two AWAP high-elevation areas. The influence of the ABI on the SHL 7-site average is the weakest amongst the different areas examined in this study. This, presumably, reflects the role of rainfall from orographic uplift in westerly streams that are not common in blocking situations. One of the major insights gained from this analysis is that the wintertime precipitation over the Snowy Mountains is most sensitive to the position of the subtropical ridge. At face value, this relationship is reasonable: as the ridge moves further south, it suppresses convection over the Snowy Mountains. A more detailed understanding of the changes in the dynamical, and possibly microphysical, processes that lead to suppressed precipitation may be a focus of future studies.
Acknowledgements
This study is supported by the Australian Research Council Linkage Project LP160101494. We are grateful to Snowy Hydro Ltd. for providing the precipitation data for the Snowy Mountains. This research did not receive any specific funding.
References
Ashok, K., Guan, Z., and Yamagata, T. (2003). Influence of the Indian Ocean Dipole on the Australian winter rainfall. Geophys. Res. Lett. 30, 1821.| Influence of the Indian Ocean Dipole on the Australian winter rainfall.Crossref | GoogleScholarGoogle Scholar |
Cai, W., van Rensch, P., Cowan, T., and Hendon, H. H. (2011). Teleconnection pathways of ENSO and the IOD and the mechanisms for impacts on Australian rainfall. J. Clim. 24, 3910–3923.
Chubb, T. H., Morrison, A. E., Caine, S., Siems, S. T., and Manton, M. J. (2012). Case studies of orographic precipitation in the Brindabella Ranges: model evaluation and prospects for cloud seeding. Aust. Meteorol. Ocean. 62, 305–321.
Chubb, T. H., Manton, M. J., Siems, S. T., and Peace, A. D. (2016). Evaluation of the AWAP daily precipitation spatial analysis with an independent gauge network in the Snowy Mountains. J. So. Hemisph. Earth 66, 55–67.
Chubb, T. H., Siems, S. T., and Manton, M. J. (2011). On the decline of wintertime precipitation in the Snowy Mountains of southeastern Australia. J. Hydrometeorol. 12, 1483–1497.
| On the decline of wintertime precipitation in the Snowy Mountains of southeastern Australia.Crossref | GoogleScholarGoogle Scholar |
CSIRO and Bureau of Meteorology (2015). Climate change in Australia information for Australia’s Natural Resource Management Regions. Technical report. (CSIRO and Bureau of Meteorology: Australia.)
Gallant, A. J. E., Kiem, A. S., Verdon-Kidd, D. C., Stone, R. C., and Karoly, D. J. (2012). Understanding hydroclimate processes in the Murray–Darling Basin for natural resources management. Hydrol. Earth Syst. Sci. 16, 2049–2068.
| Understanding hydroclimate processes in the Murray–Darling Basin for natural resources management.Crossref | GoogleScholarGoogle Scholar |
Gorman, J. D. (2003). Laboratory evaluation of the bureau designed heated tipping bucket rain gauge. 1–12 pp. Available at https://www.wmo.int/pages/prog/www/IMOP/WebPortal-AWS/Tests/ITR675.pdf [verified 5 May 2020]
Grose, M., Bhend, J., Argueso, D., Ekström, M., Dowdy, A., Hoffmann, P., Evans, J., and Timbal, B. (2015). Comparison of various climate change projections of eastern Australian rainfall. Aust. Meteorol. Ocean. 65, 72–89.
Hendon, H. H., Thompson, D. W. J., and Wheeler, M. C. (2007). Australian rainfall and surface temperature variations associated with the Southern Hemisphere annular mode. J. Clim. 20, 2452–2467.
| Australian rainfall and surface temperature variations associated with the Southern Hemisphere annular mode.Crossref | GoogleScholarGoogle Scholar |
Houze, R. A. (2012). Orographic effects on precipitating clouds. Rev. Geophys. 50, RG1001.
| Orographic effects on precipitating clouds.Crossref | GoogleScholarGoogle Scholar |
Huang, Y., Chubb, T., Sarmadi, F., Siems, S. T., Manton, M. J., Franklin, C., and Ebert, E. (2018). Evaluation of wintertime precipitation forecasts over the Australian Snowy Mountains. Atmos. Res. 207, 42–61.
| Evaluation of wintertime precipitation forecasts over the Australian Snowy Mountains.Crossref | GoogleScholarGoogle Scholar |
Jones, D., Wang, W., and Fawcett, R. (2009). High-quality spatial climate data-sets for Australia. Aust. Meteorol. Ocean. 58, 233–248.
Lewis, C. J., Huang, Y., Siems, S. T., and Manton, M. J. (2018). Wintertime orographic precipitation over Western Tasmania. J. So. Hemisph. Earth 68, 1–26.
Lu, L., and Hedlry, D. (2004). The impact of the 2002–03 drought on the economy and agricultural employment. Economic Roundup , 25–43.
Marshall, G. J. (2003). Trends in the Southern Annular Mode from observations and reanalyses. J. Clim. 16, 4134–4143.
| Trends in the Southern Annular Mode from observations and reanalyses.Crossref | GoogleScholarGoogle Scholar |
Meneghini, B., Simmonds, I., and Smith, I. N. (2007). Association between Australian rainfall and the Southern Annular Mode. International J. Climatol. 27, 109–121.
| Association between Australian rainfall and the Southern Annular Mode.Crossref | GoogleScholarGoogle Scholar |
Morrison, A. E., Siems, S. T., and Manton, M. J. (2013). On a natural environment for glaciogenic cloud seeding. J. Appl. Meteor. Climatol. 52, 1097–1104.
| On a natural environment for glaciogenic cloud seeding.Crossref | GoogleScholarGoogle Scholar |
Murphy, B. F., and Timbal, B. (2008). A review of recent climate variability and climate change in south-eastern Australia. International J. Climatol. 28, 859–879.
| A review of recent climate variability and climate change in south-eastern Australia.Crossref | GoogleScholarGoogle Scholar |
Nguyen, H., Evans, A., Lucas, C., Smith, I., and Timbal, B. (2013). The Hadley circulation in reanalyses: climatology, variability, and change. J. Clim. 26, 3357–3376.
| The Hadley circulation in reanalyses: climatology, variability, and change.Crossref | GoogleScholarGoogle Scholar |
Nicholls, N. (2005). Climate variability, climate change and the Australian snow season. Aust. Meteorol. Mag. 54, 177–185.
Nicholls, N. (2010). Local and remote causes of the Southern Australian autumn–winter rainfall decline, 1958–2007. Clim. Dyn. 34, 835–845.
| Local and remote causes of the Southern Australian autumn–winter rainfall decline, 1958–2007.Crossref | GoogleScholarGoogle Scholar |
Pepler, A., Timbal, B., Rakich, C., and Coutts-Smith, A. (2014). Indian Ocean Dipole overrides ENSO’s influence on cool season rainfall across the eastern seaboard of Australia. J. Clim. 27, 3816–3826.
| Indian Ocean Dipole overrides ENSO’s influence on cool season rainfall across the eastern seaboard of Australia.Crossref | GoogleScholarGoogle Scholar |
Pook, M. J., and Gibson, T. (1999). Atmospheric Blocking and storm tracks during SOP-1 of the FROST Project. Aust. Meteorol. Mag. 48, 51–60.
Pook, M., McIntosh, P., and Meyers, G. (2006). The synoptic decomposition of coolâ€season rainfall in the southeastern Australian cropping region. J. Appl. Meteor. Climatol. 45, 1156–1170.
| The synoptic decomposition of coolâ€season rainfall in the southeastern Australian cropping region.Crossref | GoogleScholarGoogle Scholar |
Risbey, J. S., Pook, M. J., Wheeler, M. C., and Hendon, H. H. (2009). On the remote drivers of rainfall variability in Australia. Mon. Wea. Rev. 137, 3233–3253.
| On the remote drivers of rainfall variability in Australia.Crossref | GoogleScholarGoogle Scholar |
Sarmadi, F., Huang, Y., Thompson, G., Siems, S. T., and Manton, M. J. (2019). Simulations of orographic precipitation in the Snowy Mountains of southeastern Australia. Atmos. Res. 219, 183–199.
| Simulations of orographic precipitation in the Snowy Mountains of southeastern Australia.Crossref | GoogleScholarGoogle Scholar |
Seidel, D. J., Fu, G., Randel, R. J., and Reichler, T. J. (2008). Widening of the tropical belt in a changing climate. Nature Geosci 1, 21–24.
| Widening of the tropical belt in a changing climate.Crossref | GoogleScholarGoogle Scholar |
Theobald, A., and McGowan, H. (2016). Evidence of increased tropical moisture in southeast Australian Alpine precipitation during ENSO. Geophys. Res. Lett. 43, 10901–10908.
| Evidence of increased tropical moisture in southeast Australian Alpine precipitation during ENSO.Crossref | GoogleScholarGoogle Scholar |
Thompson, D. W. J., and Solomon, S. (2002). Interpretation of recent Southern Hemisphere climate change. Science 296, 895–899.
| Interpretation of recent Southern Hemisphere climate change.Crossref | GoogleScholarGoogle Scholar |
Timbal, B. (2010). The climate of the eastern seaboard of Australia: a challenging entity now and for future projections. IOP Conference Series: Earth and Environmental Science, 012013.
Timbal, B., and Drosdowsky, W. (2013). The relationship between the decline of southeastern Australian rainfall and the strengthening of the subtropical ridge. International J. Climatol. 33, 1021–1034.
| The relationship between the decline of southeastern Australian rainfall and the strengthening of the subtropical ridge.Crossref | GoogleScholarGoogle Scholar |
Timbal, B., Arblaster, J., Braganza, K., Fernandez, E., Hendon, H., Murphy, B., Raupach, M., Rakich, C., Smith, I., Whan, K., and Wheeler, M. (2010). Understanding the anthropogenic nature of the observed rainfall decline across south-eastern Australia. The Centre for Australian Weather and Climate Research. Available at https://www.cawcr.gov.au/technical-reports/CTR_026.pdf [verified 5 May 2020].
Ummenhofer, C. C., Sen Gupta, A., Briggs, P. R., England, M. H., McIntosh, P. C., Meyers, G. A., Pook, M. J., Raupach, M. R., and Risbey, J. S. (2011). Indian and Pacific Ocean influences on Southeast Australian drought and soil moisture. J. Clim. 24, 3796–3796.
| Indian and Pacific Ocean influences on Southeast Australian drought and soil moisture.Crossref | GoogleScholarGoogle Scholar |
van Dijk, A. I. J. M., Beck, H. E., Crosbie, R. S., de Jeu, R. A. M., Liu, Y. Y., Podger, G. M., Timbal, B., and Viney, N. R. (2013). The millennium drought in Southeast Australia (2001–2009): natural and human causes and implications for water resources, ecosystems, economy, and society. Water Resour. Res. 49, 1040–1057.
| The millennium drought in Southeast Australia (2001–2009): natural and human causes and implications for water resources, ecosystems, economy, and society.Crossref | GoogleScholarGoogle Scholar |
Worboys, G. L., and Good, R. B. (2011). Caring for our Australian Alps catchments: summary report for policy makers. Department of Climate Change and Energy Efficiency, Canberra. Available at https://theaustralianalps.files.wordpress.com/2014/01/catchmentrpt2011_summary.pdf [verified 5 May 2020]