Slope and wind effects on fire propagation
Domingos X. ViegasCentro de Estudos sobre Incêndios Florestais–Associação para o Desenvolvimento da Aerodinâmica Industrial, Universidade de Coimbra, Apartado 10131, 3031-601 Coimbra, Portugal. Telephone: + 351 239 790732; fax: +351 239 790771; email: xavier.viegas@dem.uc.pt
International Journal of Wildland Fire 13(2) 143-156 https://doi.org/10.1071/WF03046
Submitted: 21 May 2003 Accepted: 9 January 2004 Published: 29 June 2004
Abstract
The vectoring of wind and slope effects on a flame front is considered. Mathematical methods for vectoring are presented and compared to results of laboratory experiments. The concept of multiple standard fire spread directions is presented. The experimental laboratory study, included effects of variable wind velocity and direction on point source flame fronts on a 30° inclined fuel bed.
Acknowledgements
The author acknowledges the help given by his students Mr E. Pires and Mr H. Sousa and by Mr Nuno Luis in the performance of the laboratory experiments and in the preliminary analysis of the data. The author thanks his colleagues Dr A. R. Figueiredo and Dr A. G. Lopes for their critical revision of the manuscript. The comments and suggestions made by anonymous reviewers of this article are also gratefully acknowledged. This work was carried out as part of the work program of the research projects INFLAME and SPREAD supported by the EU respectively under contracts ENV4-CT98–0700 and EVG1-CT-2001–00043. The support given by the Portuguese Science and Technology Foundation to this research program is also acknowledged.
Catchpole EA, de Mestre NJ , Gill AM (1982) Intensity of fire at its perimeter. Australian Forest Research 12, 47–54.
Viegas DX (2002) Fire line rotation as a mechanism for fire spread on a uniform slope. International Journal of Wildland Fire 11(1), 11–23.
| Crossref | GoogleScholarGoogle Scholar | proposed the following formulation to evaluate the rate of spread Rs and Rw , induced by wind or slope respectively, from the basic rate of spread R 0 :

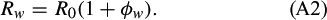
According to this formulation the modified rate of spread can be considered as the sum of the basic rate of spread and a variation induced either by slope or by wind:
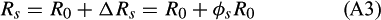
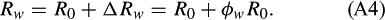
For φw and φs Rothermel presented analytical equations based on laboratory and on field experiments. The function φs depended on the slope angle α and on the porosity of the fuel bed; φw depended on a reference wind velocity and on the surface-to-volume ratio of the particles and on the fuel bed porosity as well. Both functions φw and φs are equal to zero when either wind velocity or slope angle is null.
It is easy to see that:


in which fs and fw are the corresponding functions defined by equation (6) in the present study.
The above equations (A3) and (A4) were applicable when either wind or slope acted independently from each other. For the case in which wind and slope were present simultaneously, but parallel to each other, Rothermel proposed the following formulation for the rate of spread modulus:

This equation is a generalisation of the previous ones and respects the following condition:


Equation (A7) does not consider that the rate of spread is given by the addition of the elementary rates of spread R w and Rs . Instead this equation considers the additive effect of the variations ΔRw and ΔRs .
In the presentation of the basis of the BEHAVE system, Rothermel (1983) deals with the evaluation of the local spread of sections of the fire front under arbitrary wind and slope conditions. He then proposes a vector sum of the rate of spread  induced locally by wind and the rate of spread
induced locally by wind and the rate of spread  , induced locally by slope. Although this is not specified by the author, there are indications that the modulus of each one of these vectors is given by equations (A1) and (A2), respectively. Introducing the unit vectors
, induced locally by slope. Although this is not specified by the author, there are indications that the modulus of each one of these vectors is given by equations (A1) and (A2), respectively. Introducing the unit vectors  and
and  , defining the slope and wind direction respectively, this formulation is described by:
, defining the slope and wind direction respectively, this formulation is described by:
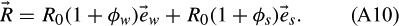
It is easy to see that, when  , this equation does not give the same result as equation (A7). In order to overcome this discrepancy, Lopes (1994) adopted the following formulation:
, this equation does not give the same result as equation (A7). In order to overcome this discrepancy, Lopes (1994) adopted the following formulation:
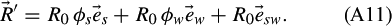
The unit vector  is parallel to the vector sum of
is parallel to the vector sum of  and
and  , as is shown in Fig. A1. This formulation is in accordance with the original equation (A7) proposed by Rothermel for the simpler case of parallel wind and slope effects.
, as is shown in Fig. A1. This formulation is in accordance with the original equation (A7) proposed by Rothermel for the simpler case of parallel wind and slope effects.
It is easy to see that the formulation expressed by equation (A11) is not suitable to be described by non-dimensional equations like those presented above for the initial formulation. Any non-dimensional form of this equation involves explicitly three parameters: β, φs and φw . Therefore it is not so convenient for a synthetic analysis. In order to compare both formulations we shall use a non-dimensional form of equations (A10) and (A11) given by:
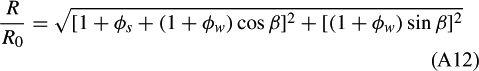
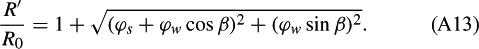
It is easy to find that the angles δ between  and
and  , and δ' between
, and δ' between  and
and  are given respectively by:
are given respectively by:

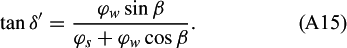
It is obvious that equations (A10) and (A11) are not equivalent as is illustrated with a particular case. If we consider β = 30°, for different values of the pair φs , φw, we obtain for each formulation the results that are shown in Table A1. In order to make the comparison easier the modulus of the rate of spread is given with reference to the basic rate of spread R 0 .
As can be seen in this table the results given by both formulations for this sample case are quite similar but nevertheless they are slightly different. The accuracy attained in the present experimental program does not permit the extraction of conclusions about the superiority of either formulation. Therefore a detailed discussion of this point is left to a future study.

|

|


