Pesticide extraction from soil into runoff under a rainfall simulator
D. Mark Silburn A B *
A B *
A Queensland Department of Environment and Science, PO Box 318, Toowoomba, Qld 4350, Australia.
B Centre for Agricultural Engineering, University of Southern Queensland, Toowoomba, Qld, Australia.
Soil Research 61(5) 468-483 https://doi.org/10.1071/SR22115
Submitted: 23 May 2022 Accepted: 11 January 2023 Published: 10 February 2023
© 2023 The Author(s) (or their employer(s)). Published by CSIRO Publishing. This is an open access article distributed under the Creative Commons Attribution-NonCommercial-NoDerivatives 4.0 International License (CC BY-NC-ND)
Abstract
Context: Runoff estimation is an important aspect of pesticide environmental behaviour and is the major loss pathway to the environment.
Aims: To improve understanding of pesticide runoff.
Methods: Data from three rainfall simulator studies was used. Twelve pesticides were studied ranged from tightly sorbed (DDE, soil sorption coefficient (KD) ~15 000 L kg−1) to weakly sorbed (dimethoate, KD < 30).
Key results: Event runoff pesticide concentrations were closely related to soil concentrations (0–25 mm depth). The ratio of runoff to soil concentration (the runoff extraction ratio, ERO), was similar for pesticides with a wide range of sorption and across the three soils: runoff concentration (μg L−1) = 28 × soil concentration (mg kg−1). ERO decreased with time after spraying, presumably due to lower concentrations in the top few mm of soil.
Conclusions: This model provides improved or similar estimates of pesticide runoff than previous models. Similar ERO values between sites was probably due to similar hydrology (high rainfall intensity, surface sealing, moist subsoils) and erosion, and because the same masses of soil and water are involved in mixing. Reduction in runoff concentrations by leaching was not influential, because infiltration was small and soil sorption too high.
Implications: Conditions studied apply during summer storms on most cotton and grain land on clay soils in the northern grain and cotton lands in eastern Australia. The model should be applicable under these conditions.
Keywords: enrichment ratios, herbicides, insecticides, partition coefficients, pesticide runoff, rainfall simulator, runoff risk, soil concentrations.
Introduction
Runoff estimation is an important aspect of the environmental fate of pesticides (Wauchope 1992). However, the pesticide runoff literature presents a seemingly random collection of concentrations, and some form of framework is needed to help understand pesticide runoff. Most studies involve only a few pesticides, so it is difficult to know if responses are due pesticide properties or the study conditions. Hornsby et al. (1996) list properties of 343 active ingredients; however, many more compounds and metabolites exist. Pesticide runoff is the outcome of a series of processes, which may be affected by pesticide properties, namely application→dissipation→leaching→partitioning→runoff extraction→sediment deposition and dillution, operating on plant canopy, crop residues and soil. Because of dissipation, runoff timing after application is important. Runoff concentrations are usually greatest in the first event after application and decline through time (e.g. Glenn and Angle 1987; Isennsee and Sadeghi 1993).
Wauchope and Leonard (1980) found ‘edge-of-field’ maximum pesticide concentrations in runoff were related to application rate, time after application and an ‘availability index’. The availability index was had classes for pesticide formulation (electrical conductivity, wettable powders, granules, etc.), solubility, and placement (foliage, soil surface or incorporated), and ranged over an order of magnitude. The model showed the importance of application rate (confirmed by Fillols et al. (2020)) and time after application (dissipation). So as a minimum, a model for pesticide runoff should consider the application rate and dissipation rate.
What predictive performance can we expect from pesticide runoff models? Singh and Jones (2002) found that the PRZM model could predict pesticide runoff within an order of magnitude of measured data, with better agreement for larger events. With improved hydrologic calibration, agreement was within a factor of 3. Young and Fry (2019) found pesticide runoff could be modelled within a factor of two at the high-end of the runoff measurements where the most influential high mass-load events occurred, after optimisation of model parameters. Connolly et al. (2001) used the GLEAMS model to predict pesticide runoff loads using parameters from the literature and optimisation and could model pesticide runoff loads to within a factor of two of the measured data.
Leonard et al. (1979), Spencer et al. (1985) and Baker (1980) found strong relationships between pesticide concentrations in surface soil and in runoff. This paper focuses on this relationship and how different pesticides behave. These data are contrasted with data from catchments and rainfall simulator plots the literature in Silburn (2003). The objective is to determine the relationship between pesticide concentrations in soil and the resulting concentrations in runoff when rainfall is applied using a rainfall simulator, thereby starting to define more general relationships of this type.
Conceptual framework
Runoff extraction from soil
During rainfall, chemicals are leached downwards depending on the partitioning coefficient, reducing surface soil concentrations. Then, extraction into runoff occurs, in water and sediment. The amount of chemical available for leaching and extraction depends on the soil concentration in the ‘runoff-mixing layer’. Runoff concentrations are highly related to soil concentrations for pesticides (Leonard et al. 1979; Baker 1980; Spencer et al. 1985) and soluble phosphorous (P) (Sharpley et al. 1982). Soluble chemical extraction into runoff is greatest at the surface and decreases exponentially with depth, with some extraction from 20 mm (Ahuja and Lehman 1983). Solute and sediment-bound extraction exhibit similar responses to rainfall intensity and energy, cover, infiltration and slope etc. (Ahuja 1986, 1990). Thus, there is a positive correlation between solute and sediment extraction (Sharpley 1985). For suspended sediment, measured concentrations of strongly sorbed chemicals are generally 1–10 times the soil concentrations (Leonard et al. 1979; Willis et al. 1983) because of size-selective erosion (enrichment). Also, the chemical distribution with depth in the soil is important but is generally unknown.
Concentrations of weakly sorbed chemical in the mixing-layer decrease exponentially during rain (Baker 1980). Truman et al. (1998) confirmed this for pesticides. When leaching is restricted, dilution of the surface concentration into runoff occurs. Runoff concentrations are one to two orders of magnitude greater than where leaching is unrestricted (Ahuja and Lehman 1983). Snyder and Woolhiser (1985) observed significant exfiltration on sloping flumes (‘interflow’), which increased removal of dye in runoff. Where leaching was restricted by less permeable subsoil, dissolved chemicals in runoff are contributed by interflow and surface extraction. Barnett et al. (1972) found runoff concentrations were highest for nutrients (e.g. nitrogen, potassium, chlorine) for interflow rather than overland flow. However, Edwards et al. (1980) observed lower glyphosate runoff concentrations from interflow than overland flow, due to adsorption. Thus, chemicals need to be weakly sorbed to be transported in leaching and interflow.
Runoff extraction ratios
Pesticide event runoff concentrations (CRO μg L−1) are related to soil concentrations (Leonard et al. 1979):
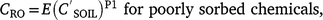
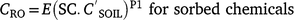
where is soil concentration (mg kg−1), SC is sediment concentration1 (kg L−1), E is extraction coefficient, and P1 is fitted coefficient.
Leonard et al. (1979) conceptualised E as an ‘extraction coefficient’ for weakly sorbed pesticides and as an ‘enrichment factor’ for sorbed pesticides. Exponent P1 represents non-linearity and is slightly less than 1.0 for sorbed pesticides: 0.83 Leonard et al. (1979), indicating a changing sediment composition with time. P1 is slightly greater than 1.0 for poorly sorbed pesticides: 1.2 Leonard et al. (1979) and 1.03 Baker (1980), reflecting changing extraction efficiency and pesticide distribution in surface soil with time. Assuming linearity (P1 = 1), runoff extraction ratio is
. ‘Maximum potential runoff concentration‘ is where runoff has a concentration equal to the pesticide mass in the soil divided by rainfall volume, defining the upper bound for runoff extraction. For 50 mm of rain, the maximum runoff extraction ratio is 300 for 0–10 mm soil and 30 for 0–2 mm soil (bulk density 1500 kg m−3).
Materials and methods
Experimental outline
Pesticide soil concentrations (0–25 mm) before rain and runoff concentrations from rainfall simulator plots at three sites were used. At one site (Gatton), a range of times after application and cultural treatments (e.g. range of cover, plot sizes and slopes, rainfall intensity and spray formulation, were studied. As treatments other than cover and time after pesticide application made no difference to runoff concentrations, at later studies (Emerald and Jondaryan), only time after applications (both) and a range of cover (Emerald) were studied. Also, prior wheel traffic was included as a treatment at Emerald and Jondaryan as it occurs in all cotton fields, and has a large effect on runoff and pesticide losses. Sites, rainfall simulator, pesticide application and analysis methods are described by Silburn (2003) and Silburn et al. (2002, 2013). Pesticides with a wide range of properties were studied.
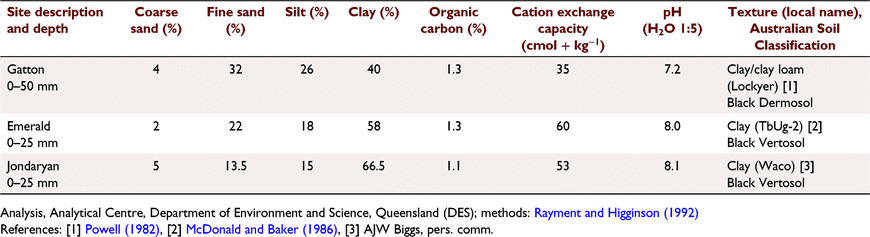
Locations and soils
Rainfall simulator studies were run at sites in Fig. 1 and Table 1:
-
Gatton, University of Queensland, on an alluvial levee (27°32.173′S, 152°20.052′E). Soil has a dark clay loam to light clay (crusting or cloddy) surface, Black Dermosol (Isbell 2002) used for cropping.
-
Emerald irrigated cotton farm west of Emerald, Queensland (23°31.6′S, 148°9.3′E), on a Black Vertosol (Isbell 2002), strongly self-mulching and cracking. Used for irrigated cotton for >20 years.
-
Jondaryan, Queensland (27°25′50″S 151°37′40″E), on the gently undulating alluvial plain of Oakey Creek. Soil is a Haplic self-mulching Black Vertosol (Isbell 2002) used for irrigated cotton and winter cereals.
|
Hill-furrow geometry and plot conditions
Studies were conducted on row-crop layouts with 1 m rows, hills 0.25 m high, linear 50% side-slopes 0.4 m long, except Gatton (0.75 m rows, 50% side-slopes). Furrows are used for irrigation and wheel traffic. Downfield slopes were 0.2–1.5% (Table 2). Studies were conducted early in the cotton season between planting and first irrigation. All sites had low cover (<5%), except where cover was applied. Surface soils (0–50 mm) were loose and at air-dry moisture content; the surface had a strong crust at Jondaryan. There were no cracks, having been pre-irrigated or fallowed. All sites had a few cm of loose soil in the furrow over firm moist subsoil, compacted to varying degrees. Gatton was run in April with no crop and wheel traffic was random. Emerald and Jondaryan had a wheel track and non-wheel track as separate plots under the simulator.

|
Rainfall simulator
The rainfall simulator, described by Loch et al. (2001), uses 13 in-line oscillating flat fan Veejet 80 100 nozzles spraying downwards, wetting an area 13 m long and 2.5 m wide. Rain was applied for 40 min or more, at 95 mm h−1 (Gatton and Emerald) and 70 mm h−1 (Jondaryan) (Table 2). Intense storms were applied as they cause most soil loss in this environment (Wockner and Freebairn 1991). Plot conditions and hydrology are summarised in Table 2.
Runoff, sediment, and pesticide measurements
Runoff rates and sediment concentrations were measured every 2 min. Flow-weighted mean pesticide concentrations in runoff were measured and averaged for the two plots to be compatible with soil concentrations (0–25 mm), which were averaged for hill and furrow. Pesticide concentrations in water and sediment were calculated from filtered flow-weighted composite samples taken through hydrographs and were used to calculate partition coefficients. A range in soil concentrations was obtained by including a range of times after spraying and banded and blanket sprays, giving four orders of magnitude in soil concentrations. Bulk density was measured after rain and used to convert soil concentrations (mg kg−1) to loads in g of active ingredient per ha (g.a.i. ha−1).
Pesticide treatments and analysis
Pesticides studied and their properties
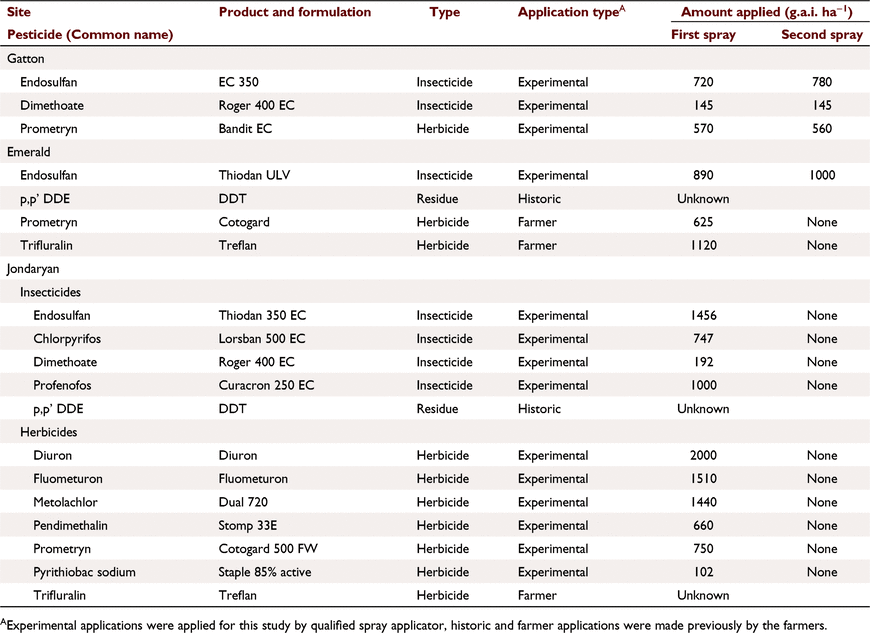
Endosulfan (α-, β-isomers), endosulfan sulfate (toxic breakdown product) (the sum called total endosulfan) and prometryn were studied at all sites. Other pesticides were applied as experimental treatments or were present in soil (Table 3).
|
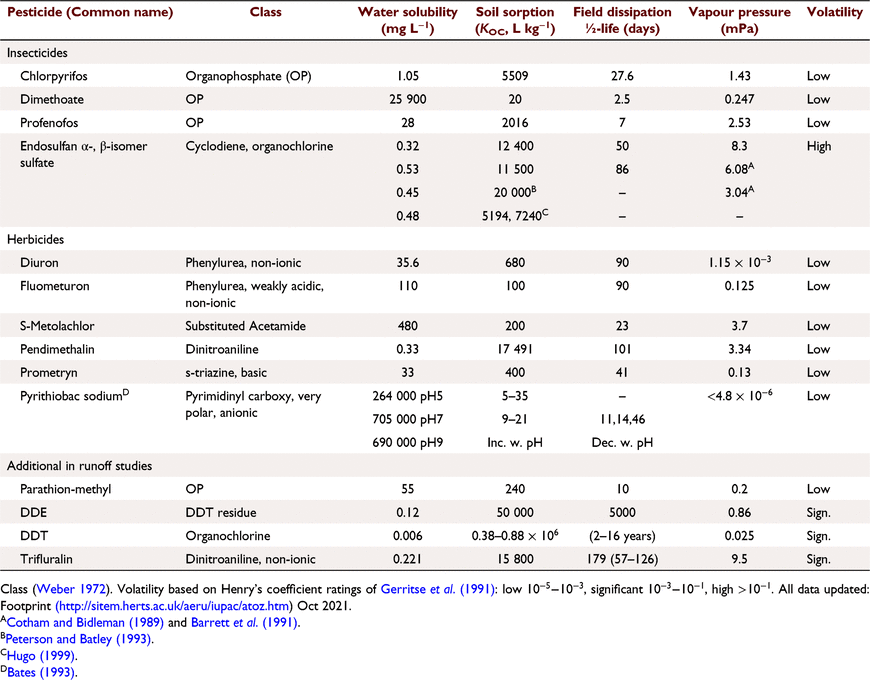
The 12 pesticides and their published properties (Table 4) are:
-
Dichlorodiphenyldichloroethylene (DDE): low water solubility (0.003 mg L−1) and high KOC (380 000–880 000 L kg−1),
-
Endosulfan, trifluralin, chlorpyrifos and pendimethalin: low solubility (0.3–0.4) and higher KOC (8000–12 400 L kg−1), and profenofos (Sol 28, KOC 5000 L kg−1),
-
Diuron, prometryn, metolachlor and fluometuron (Solubility in water (mg L−1) (Sol) 33–530): low KOC (100–480 L kg−1).
-
Dimethoate (Sol ~40 000 (mg L−1), KOC 20 L kg−1) and pyrithiobac sodium (Sol ~700 000 (mg L−1), KOC 9–21 L kg−1), soluble and weakly sorbed.
|
Pesticide and cultural treatments
Gatton
The main treatment was time after spraying (ranging from 2 h to 15 days) with two applications 6 days apart. Endosulfan, prometryn and dimethoate were blanket sprayed on: (1) four pairs of plots (emulsified concentration (EC) formulation, bare, 1.6 m long, 1.5% slope, 95 mm h−1 rain) and rain was applied 2 h after the first spray and 2 h, 26 h and 9 days after the second spray; and (2) five pairs of plots of secondary treatments where one variable was altered, with rain 2 h after the first spray: plot length (12 m), slope (0.9%, 4.3%), cover (100%), rain 2 h after two sprays, formulation (ultra-low volume ULV); second storm on 12 m plots, 20 min after the first. There was some variation in runoff (30–41 mm), infiltration (22–28 mm) and sediment concentration (24–57 g L−1). Stubble covered plot gave double the infiltration.
Emerald
Plots had a range of cover (wheat stubble or cotton trash), with and without wheel traffic. Rain was applied 4–7 days after two endosulfan applications, 17 days after prometryn (banded) and 50 days after trifluralin application. Data were averaged for traffic treatments and pooled as ‘bare’ (bare/cotton trash, cover 0–10%, five pairs of plots) and ‘covered’ treatments (30–50% cover, three pairs of plots). There were no significant differences in pesticide runoff concentrations between treatments within these groups, or due to time after spraying. Data for nutrients (N and P species) in soil and runoff, particularly nitrate (NO3-N) (Silburn and Hunter 2009), are presented as a tracer of dissolved chemicals.
Jondaryan
The focus was to create a range in soil concentrations. Five simulations (pairs of plots) were run: three blanket plots sprayed at 5, 25 and 34 days and two banded plots 2.3 and 34 days before rain. Four insecticides and six herbicides were applied (Table 3). Trifluralin and DDE were present from past applications (>1 year).
Pesticide application
Pesticides (Table 3) were applied using hand applicators with EC (high volume 50 L ha−1) or ULV (3–4 L ha−1) emitters, by professional applicators. Rates were confirmed by soil sampling soon after application.
Sampling for pesticide analysis
Two types of ‘runoff samples‘ were taken:
-
rainwater daily and runoff (~500 mL) at five times during the hydrograph, analysed for total pesticide concentrations (‘hydrograph’),
-
composite runoff samples during the hydrograph, filtered and analysed for pesticide concentrations in water and sediment phases separately, to determine partitioning. A runoff portion was added to a single bottle, with sampling duration kept constant, providing a flow weighted sample (‘bulked’) (Masters et al. 2013).
Hydrograph and bulked concentrations are compared in Supplementary material A.
‘Soil samples‘ were taken (0–25 mm) from hill and furrow separately, from eight locations on the plot, composited and mixed, using a vertically sided trowel (70 mm wide by 110 mm long) of 25 mm depth. The trowel and sampling container were cleaned with methanol between uses to prevent contamination. At Emerald, ‘crop residues‘ sub-samples were taken from a known area and 0–25 mm soil taken from these areas, so soil samples did not contain pesticides intercepted on crop residues. Crop residue mass per unit area was measured, to convert concentrations (mg kg−1) to loads (g ha−1) and to determine total pesticide load. Pesticide analysis samples were placed in glass jars with Teflon seals, into insulated boxes with ice and sent to the laboratory by air courier at 4°C. Filtering and extraction of water samples commenced within 1 day. Soil and trash samples were stored at −15°C.
Pesticide analysis
Runoff, rainwater, and soil were analysed for the pesticides in Table 4.
For runoff samples, initial phase of analysis was different for hydrograph and bulked samples:
-
Hydrograph samples and rainwater were analysed for total pesticide concentrations. The sediment portion was extracted by refluxing with dichloromethane/acetone (1:1) and added to the water portion and extracted with dichloromethane and hexane.
-
Bulked runoff samples were filtered (0.7-μm glass fibre) and analysed for sediment-sorbed and soluble pesticides separately. The water portion was extracted by shaking with dichloromethane and hexane separately. Sediment was extracted by refluxing with a 1:1 mixture of dichloromethane and acetone, reduced to a small volume, added to 500 mL distilled water, extracted with dichloromethane and hexane.
Runoff samples from Gatton and Emerald were analysed by Pesticides Laboratory, Indooroopilly. Following extraction, pesticides were partitioned into hexane and cleaned up on a Florisil column and determined using gas chromatography (GC) with ECD (electron capture detector), NPD (nitrogen phosphorous detector) and mass spectrophotometry (MS), using a 30 m DB-1 capillary column. Jondaryan water samples, other than pyrithiobac sodium, were analysed by Queensland Health Scientific Services (QHSS), using standard multi-residue GC and HPLC methods (Supplementary material B). Pyrithiobac sodium in soil, water and sediment was analysed by Analchem Bioassay using GC-MS (Bruns and Tauber 1992; Sumpter et al. 1996).
Samples for soil and crop residue were analysed for pesticides (Table 4) as follows: for Emerald and Gatton, by the Pesticides Laboratory, Indooroopilly. Pesticides were extracted with methanol/water (4:1) and partitioned into hexane and cleaned up on a Florisil column and determined using GC with ECD, NPD and MS and a 30 m DB-1 capillary column; for Jondaryan, soil pesticides other than pyrithiobac sodium were analysed by QHSS, using standard multi-residue GC and HPLC methods. Pyrithiobac sodium in soil was analysed by two methods described in Supplementary material C.
Statistical analysis
Two analyses were used: (1) analysis of variance (ANOVA) using general linear models, was used to determine differences between treatment means; and (2) regression was used to examine how well soil concentrations explained variance and trend in pesticide runoff concentrations, and whether there were differences for pesticides. Mean extraction ratios calculated by ANOVA and regression slope were different, due to different weighting of data. Extraction ratios calculated by regression, with a least squares weighting, are preferred. Regression analysis, with grouping for pesticides, was used to describe effects of slope, infiltration amount, sediment concentration, days since spraying and cover on extraction ratios.
Results and discussion
Before pesticide applications, soils contained no detectable residues, except minor amounts of endosulfan sulfate at Emerald.
Gatton
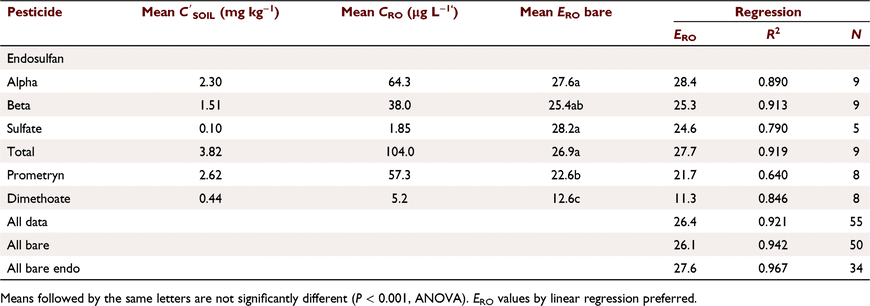
Pesticide runoff concentrations were closely related to soil concentrations (Fig. 2a), CRO (μg L−1) = 26.1 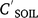 (mg kg−1) (R2 = 0.937, N = 50, P < 0.001) for bare plots. ERO was similar (not statistically different, n.s.d.) for endosulfan compounds (Table 5), including sulfate (Fig. 2b). Dimethoate and prometryn had significantly lower runoff extraction (Table 5), with ERO = 11.4 and 21.7, respectively. Dimethoate extraction was lower in both sediment and water; dimethoate concentrations in the runoff-mixing layer were lower than indicated by soil concentrations due to rapid dissipation (Silburn 2003) and leaching, consistent with dimethoates lower partitioning. However, dimethoate and other organophosphates had high extraction (37) at Jondaryan, even though they also dissipated rapidly. Formulation, plot length, rainfall intensity (95–125 mm h−1), runoff (30–41 mm), infiltration (22–30 mm), sediment concentration (24–57 g L−1), slope (0.9–4.3%) and event number had no significant effect on runoff extraction (Fig. 2).
(mg kg−1) (R2 = 0.937, N = 50, P < 0.001) for bare plots. ERO was similar (not statistically different, n.s.d.) for endosulfan compounds (Table 5), including sulfate (Fig. 2b). Dimethoate and prometryn had significantly lower runoff extraction (Table 5), with ERO = 11.4 and 21.7, respectively. Dimethoate extraction was lower in both sediment and water; dimethoate concentrations in the runoff-mixing layer were lower than indicated by soil concentrations due to rapid dissipation (Silburn 2003) and leaching, consistent with dimethoates lower partitioning. However, dimethoate and other organophosphates had high extraction (37) at Jondaryan, even though they also dissipated rapidly. Formulation, plot length, rainfall intensity (95–125 mm h−1), runoff (30–41 mm), infiltration (22–30 mm), sediment concentration (24–57 g L−1), slope (0.9–4.3%) and event number had no significant effect on runoff extraction (Fig. 2).
|
ERO decreased with days since last spray (DSS2) for α-, β- and total endosulfan (ranging from 27 at 2 h to 21 at 9 days) and prometryn (23–18). ERO was constant for dimethoate and increased for endosulfan sulfate (ERO = 26.55 + 0.787. DSS2, R2 = 0.819, P = 0.008). α-, β- and total endosulfan were similar (n.s.d.): ERO = 28.1–0.791. DSS2 (R2 = 0.87, P < 0.001). Prometryn had a similar slope (n.s.d.) but lower constant due to lower extraction. Reduction in ERO with time is probably due to changes in the distribution in the soil surface. Increase in ERO for endosulfan sulfate was due to conversion of α- and β- to sulfate. There was no relationship between ERO and runoff KP.
Emerald
Total runoff concentrations for bare plots were strongly related to soil concentrations before rain (Fig. 3), CRO = 27.9 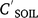 (R2 = 0.84, P < 0.001) for all endosulfan compounds and prometryn (n.s.d., Table 6) even though prometryn has a much lower KP and was band sprayed. Similar runoff extraction for prometryn is inconsistent with result for Gatton; a small (though significant) difference for prometryn at Gatton and result here indicates runoff extraction of prometryn is like endosulfan from a practical standpoint.
(R2 = 0.84, P < 0.001) for all endosulfan compounds and prometryn (n.s.d., Table 6) even though prometryn has a much lower KP and was band sprayed. Similar runoff extraction for prometryn is inconsistent with result for Gatton; a small (though significant) difference for prometryn at Gatton and result here indicates runoff extraction of prometryn is like endosulfan from a practical standpoint.
|
|

|
ERO values were significantly lower for DDE and trifluralin than for endosulfan and prometryn (Table 6, Fig. 3). DDE and trifluralin have are more tightly sorbed and dominantly transported in sediment; DDE KP = 10 000, 99% in sediment, trifluralin KP = 720, 92%. DDE and trifluralin concentrations in sediment (mg kg−1) were lower than in 0–25 mm soil, particularly for trifluralin (Table 6). Thus, concentrations were lower in the surface few mm of soil than in 0–25 mm soil. This is probably due to their volatilisation, which is rated as significant (Table 4). In contrast, endosulfan concentrations in sediment were 1–2 times those in 0–25 mm soil and probably had an increased concentration towards the soil surface. Trifluralin had more variable runoff extraction than other (Fig. 3b); regression analysis indicated no relationship between runoff and soil concentrations (Table 6). This may be related to incorporation of trifluralin being haphazard and volatilisation, creating spatial variability in concentration gradients in the soil.
Comparison with N and P species
N and P runoff concentrations had a lower relationship to soil concentrations than pesticides (Fig. 4). Oxidised N is comparable as both soil and runoff analysis are NO3-N. NO3-N is easily leached and its presence in runoff indicates leached chemicals return to the surface due to ponding on the subsoil (i.e. interflow).
Jondaryan
Pesticide runoff concentrations at Jondaryan were highly related to soil concentrations before rain (Fig. 5), CRO = 30.4 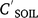 (R2 = 0.91, P < 0.001). Soil and runoff concentrations of metolachlor, fluometuron, diuron and prometryn were 1–2 orders of magnitude greater than pyrithiobac sodium (applied ~1/10th lower rate) and trifluralin, but runoff extraction was similar (Fig. 5). Similarly, endosulfan soil and runoff concentrations were considerably greater than dimethoate, chlorpyrifos and profenofos, which dissipated much more rapidly (Silburn 2003), or DDE, but runoff extraction was similar (Fig. 5b).
(R2 = 0.91, P < 0.001). Soil and runoff concentrations of metolachlor, fluometuron, diuron and prometryn were 1–2 orders of magnitude greater than pyrithiobac sodium (applied ~1/10th lower rate) and trifluralin, but runoff extraction was similar (Fig. 5). Similarly, endosulfan soil and runoff concentrations were considerably greater than dimethoate, chlorpyrifos and profenofos, which dissipated much more rapidly (Silburn 2003), or DDE, but runoff extraction was similar (Fig. 5b).
ERO values were not significantly related to slope, rainfall intensity, runoff amount or sediment concentration. ERO values decreased with increasing infiltration (R2 = 0.294), by 5.9 across the range of infiltration (15–20 mm), due to a similar decrease in water phase extraction (R2 = 0.591), with no effect on sediment phase extraction (Table 7). There was no relationship between ERO and runoff KP.
|
Contrast of herbicides and insecticides
Herbicides had higher runoff extraction (ERO 31.9) than insecticides (21.7) (R2 = 0.927, P < 0.001 for difference in slope). Thus, for a soil concentration of 1 mg kg−1, runoff concentration was 32 for herbicides and 22 μg L−1 for insecticides, not a large practical difference. However, herbicides had significantly different runoff extraction2, trifluralin (37.1), diuron (30.6), prometryn (28.6), metolachlor (27.0), and other herbicides (fluometuron, pyrithiobac sodium, pendimethalin) ERO = 20–22 (like insecticides). Among insecticides, ERO was higher for OP’s (24–26), endosulfan sulfate (23.1), total endosulfan (19), β-endosulfan (18), DDE (17) and α-endosulfan (16). Again, ERO did not relate to KP. Overall, runoff extraction was n.s.d. between pesticides. (see footnote 2).
Runoff extraction was less efficient with greater time since spraying. When pesticides were sorted into groups that were n.s.d. (R2 = 0.594, P < 0.001):


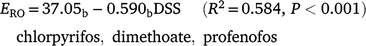

Subscripts denote the last two groups had significantly higher constants than the first two groups (P < 0.001). OP values had a significantly greater slope (P = 0.004), consistent with rapid dissipation. Decrease in ERO with DSS was due to reduced water phase extraction (Table 7). Sediment extraction had no trend with DSS. However, sediment (mg kg−1) was significantly lower at two DSS. Banded and blanket plots had similar runoff extraction (n.s.d., Table 7). Averaging hill and furrow concentrations accounted for band spraying.
Cover effects on runoff extraction
Where endosulfan was applied over cover and rain applied 2 h later at Gatton, runoff extraction was like that for bare soil for total, α-, β- and endosulfan sulfate (n.s.d., Fig. 2). Washoff from cover was not a source of these pesticides in runoff. The majority of pesticide washoff from cover occurs in the first 5–15 mm of rainfall (Dang et al. 2016). In contrast, prometryn and dimethoate had higher runoff extraction from cover, indicating washoff from cover contributed; they are less strongly sorbed than endosulfan and may be less sorbed on wheat stubble. Greater infiltration with cover (50 c.f. 22–30 mm for bare plots) did not lead to lower runoff extraction if leaching from the runoff-mixing layer was significant; interflow probably occurred. Even though runoff extraction was similar or increased with cover, runoff concentrations were considerably lower (except prometryn), due to volatilisation from cover.
At Emerald, ERO values were lower with cover but were only significantly different for endosulfan sulfate and DDE (Table 8). For endosulfan sprayed on cover, a major effect of cover was to reduce soil concentrations presumably by volatilising considerable endosulfan, with runoff concentrations reduced accordingly. This outweighs other effects of cover, but runoff extraction was still 30% lower than from bare soil, due to less sediment transport.
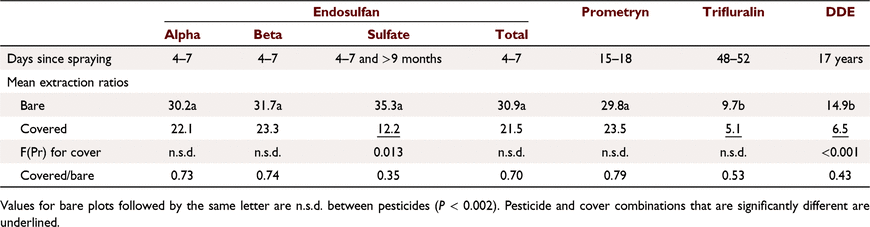
|
For other pesticides at Emerald, which were in the soil under the cover, response to cover depended on sorption; ERO was reduced most for more sorbed trifluralin and DDE and least for prometryn (Table 8). Prometryn concentrations were similar between cover treatments in both soil and runoff. Because prometryn was transported mainly in water, effect of cover on sediment loss had little impact. ERO values were lower for cover for endosulfan sulfate, trifluralin and DDE. For these more strongly sorbed compounds, a large proportion was transported in sediment. Cover explained 61% of the variance in ERO (P < 0.001), with similar slopes (n.s.d.) and different intercepts (trifluralin < DDE < others). ERO values were also positively correlated with sediment concentration (R2 = 0.68) and negatively correlated with infiltration (R2 = 0.57). Sediment concentration and infiltration were closely related to cover (Silburn and Glanville 2002). ERO response to infiltration is probably spurious, reflecting the cover effect on sediment concentration.
Of factors that were affected by cover, infiltration and sediment transport (Silburn and Glanville 2002), which affect runoff extraction, influence of sediment dominates and runoff extraction varies with sorption. However, infiltration matters for poorly sorbed chemicals; NO3-N runoff concentrations increased with increasing cover, particularly for wheel tracks due to interflow.
General discussion
There was a high degree of consistency in runoff extraction for pesticides with a wide range of properties. Runoff concentrations are mainly determined by surface soil concentrations. Runoff extraction in rainfall simulation studies were also consistent with literature data (Silburn 2003; Silburn and Kennedy 2007; Thorburn et al. 2013). However, there are exceptions such as trifluralin and DDE (Emerald) and dimethoate (Gatton), where ERO values were lower. For dimethoate, this was associated with rapid dissipation and leaching. This did not occur for OP’s at Jondaryan where infiltration was lower, and less leaching occurred. For trifluralin and DDE, it was associated with higher sorption and insufficient sediment transported.
In the introduction, it was noted that models could predict pesticide runoff loads to within a factor of two of the measured data (Connolly et al. 2001; Young and Fry 2019). Here, relationships for measured pesticide runoff concentrations plotted against soil concentrations had R2 of 0.94 at Emerald (Fig. 2), 0.92–9.94 at Gatton (Table 5), and 0.94–0.97 at Jondaryan (Fig. 5), or a fit of less than one half of an order of magnitude (albeit with some data that did not fit as described above) over a four order of magnitude range in soil and runoff concentrations. These relationships have also been shown to be strong in other papers in the literature (Leonard et al. 1979; Baker 1980; Spencer et al. 1985; Melland et al. 2016; Silburn et al. 2023). Several papers (Silburn 2003; Silburn and Kennedy 2007; Thorburn et al. 2013) have shown that the relationships in these papers and those presented here are also consistent to within an half an order of magnitude. The strong relationships found here and in the literature are probably due to the small scale of the catchments studied (a few square metres to some hectares). These results reinforce the directness of the relationship between concentrations in soil and those measured in runoff. What differentiates runoff extraction between pesticides?
Different runoff extraction for bare soil occurs if:
-
Pesticides are differentiated by leaching, requiring different sorption and significant infiltration, and that leached pesticides were not returned to runoff in interflow, discussed in the section ‘The role of leaching’.
-
Pesticides are differentiated by sorption, requiring different sorption and low sediment concentrations, wherein poorly sorbed pesticides are extracted into runoff, but strongly sorbed pesticides are absent. With higher sediment concentrations, pesticide concentrations only depend on soil concentration, discussed in the section ‘Why pesticides had similar runoff extraction’.
-
Pesticides have different concentration-depth distributions in the runoff-mixing layer. Recently sprayed pesticides had a higher concentration in the upper few mm of soil compared to the average over the sampled depth, and runoff extraction is greater. Over time, greater dissipation near the surface and downward movement leads to lower concentrations in the surface and lower runoff extraction. Pesticides prone to volatilisation and photolysis also have a lower concentration in the surface.
The role of leaching
Concentration reductions in the runoff-mixing layer by leaching can be calculated using the advection equation (Leonard et al. 1987):
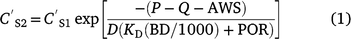
where 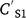 and
and  are soil concentration (mg kg−1) before and after rain (mm) and runoff Q (mm), AWS is available water storage (mm), D is soil depth (mm), KD is sorption coefficient (L kg−1), BD is bulk density (kg m−3), and POR is soil porosity (v v−1).
are soil concentration (mg kg−1) before and after rain (mm) and runoff Q (mm), AWS is available water storage (mm), D is soil depth (mm), KD is sorption coefficient (L kg−1), BD is bulk density (kg m−3), and POR is soil porosity (v v−1).
Leaching effects on soil concentrations (0–25 mm) for various KD values and pesticides, calculated with Eqn 1, are illustrated in Fig. 6. For infiltration on bare plots at Emerald (49 mm), soil concentrations are barely affected by leaching for pesticides with KD > 100 (e.g. endosulfan, DDE), even with 100 mm of infiltration. Concentrations are reduced by 10% during 50 mm of infiltration for KD = 14 (prometryn). With KD = 1.4 (atrazine, fluometuron), concentration is reduced by 25% with 30 mm of infiltration and 50% with 50 mm. KD needs to be ~0.2 for soil concentration to halve with 30 mm of infiltration. Thus, KD < 2 is needed for leaching to significantly reduce the concentration in 0–25 mm soil. Runoff partition coefficients were generally greater than 10 and only <5 for a few pesticides (Silburn 2003) and then only for a few days after spraying.
|
|
At other simulator sites, infiltration amounts (17–25 mm) were half those at Emerald (Table 2). Infiltration only exceeded the AWS (0–25 mm soil) at runoff commencement; considerable pesticide was available for runoff mixing. Where infiltration was greatest (65 mm Emerald with cover), NO3-N runoff indicates interflow occurred (Silburn and Hunter 2009), consistent with greater runoff Br− concentrations where leaching was restricted (Ahuja and Lehman 1983). However, prometryn concentrations (less sorbed) only increased slightly on covered wheel tracks because some prometryn was adsorbed. Thus, it appears that pesticides must be very weakly sorbed to respond like NO3-N and Br.
A case where leaching did reduce runoff extraction was dimethoate at Gatton where runoff extraction more than halved. Soil concentrations after rain were 5% of concentrations before rain, whereas endosulfan was not affected. In dissipation studies (Silburn 2003), downwards movement into 25–50 mm soil occurred at Jondaryan under natural rainfall for a small proportion of endosulfan and various herbicides. For herbicides, proportion moved down increased with lower sorption. This occurred over weeks with 140 mm rainfall in 62 days. Thus, reduction in runoff concentration by leaching was rarely influential in the simulator studies; infiltration was small and soil sorption too high. However, leaching may be important for dissipation for soluble compounds in the long term, with higher rainfall. Infiltration depth needs to be considerably larger and KD considerably smaller for leaching to influence runoff concentrations.
Infiltration rates on the black Vertosol at Emerald (31–46 mm h−1 wheel track and 44–54 mm h−1 non-wheel tracks) exceed final infiltration rates (5–35 mm h−1) measured by Loch and Foley (1994). The black Vertosol at Jondaryan had least infiltration, but most cropping soils in the region have similar or lower infiltration. When subsoil is moist, infiltration rate are 10–25 mm h−1 or less (Loch and Foley 1994; Connolly et al. 1997a) because of restricted infiltration through compacted subsoils (Silburn and Connolly 1995). Thus, hydrologic conditions here are typical of large areas of agricultural land after fallowing or pre-irrigation.
Why pesticides had similar runoff extraction
Partition coefficients in simulator studies varied over 4–5 orders of magnitude (Silburn 2003). It is intriguing that a fixed proportion of soil concentrations were extracted into runoff. Similarity of runoff extraction ratios across the range of KP values depends on sediment concentrations being in a certain range. Several factors push runoff extraction towards similarity rather than difference, so long as leaching is restricted. An important factor is that the soil mass and water volume involved in mixing are the same for all pesticides on a plot and between plots and soils. Many factors that increase solute extraction also increase sediment detachment (Ahuja 1986, 1990). Other mitigating factors are:
-
As sediment concentration decreases, physical enrichment of sediment increases, so that sediment phase concentrations do not decrease in proportion to sediment concentration. The net effect is to moderate effects of sediment concentration on runoff extraction, except at very low or much higher sediment concentrations, where runoff extraction will become different depending on sorption (see Spencer et al. (1985) in Silburn (2003)). Thus, for Emerald, where cover reduced sediment concentration from 20 to 6 g L−1, ERO for DDE and trifluralin halved while the ERO for prometryn was not reduced.
-
Pesticides were extracted in both water and sediment phases, except for DDE.
Similar runoff extraction for simulator studies was also because of similar infiltration and erosion. Infiltration was low, due to surface sealing, compacted and moist subsoil. Sites had low slopes (0.2–2%), and similar erosion/deposition operated, although sediment concentration varied from 10 to 60 g L−1. Higher runoff extraction in the literature (ERO ~ 80–200, Silburn (2003)) were related to greater erosion.
Conclusions
Pesticide runoff concentrations for 12 pesticides from three simulated rainfall studies were compared with their soil concentrations before rain. Total pesticide runoff concentrations were closely related to soil concentrations (0–25 mm) with three exceptions. Dimethoate was leached from the runoff-mixing zone at Gatton, while trifluralin and DDE are tightly sorbed and too little sediment was transported. Otherwise, runoff extraction was similar for pesticides with a wide range of sorption and three soils: runoff concentration (μg L−1) = 28 × soil concentration (mg kg−1). Runoff extraction ratios decreased with time after spraying, presumably due to lower concentrations in the top few mm of soil. Similar runoff extraction between sites was due to: (1) similar infiltration and erosion; (2) interflow and ineffective leaching; and (3) sediment concentrations were high enough to ensure transport of strongly sorbed pesticides. Without significant leaching and with sufficient sediment transport, little differentiation in runoff extraction occurred for pesticides of widely different partitioning. Where hydrologic and erosion differed markedly from conditions studied here, runoff extraction will differ, and pesticides will be differentiated by sorption. Conditions studied occur on much of the grain and irrigated cotton lands on clays in the northern grain and cotton lands in eastern Australia.
Supplementary material
Supplementary material is available online.
Data availability
The data that support this study will be shared upon reasonable request to the corresponding author.
Conflicts of interest
The authors declare no conflicts of interest.
Declaration of funding
Funding was provided by Cotton Research and Development Corporation and Murray Darling Basin Commission, and the Department of Environment and Science.
CRediT authorship contribution statement
Sole author undertook all conceptualisation, data curation, formal analysis, funding acquisition, investigation, and writing. Jenny Foley (Department of Resources, Queensland) undertook statistical analysis.
Acknowledgements
Jenny Foley (QDR) ably performed statistical analysis. Many people contributed to field work; main contributors were Steve Glanville and Jim Bourke (QDR). Laboratory pesticide analysis was supervised by Bruce Simpson (Qld Department of Primary Industries) and Mary Hodge (Qld Health). Drs Stephen Lewis (James Cook University) and Francisco (Paco) Sanchez-Bayo (University of Sydney) provided very useful reviews. Prof. Ivan Kennedy is thanked for thorough and gentle PhD supervision and never, ever having a wallet at lunch!
References
Ahuja LR (1986) Characterization and modeling of chemical transfer to runoff. In ‘Advances in soil science. Vol. 4’. (Eds BA Stewart) pp. 149–188. (Springer) https://doi.org/10.1007/978-1-4613-8612-4_3Ahuja LR (1990) Modeling soluble chemical transfer to runoff with rainfall impact as a diffusion process. Soil Science Society of America Journal 54, 312–321.
| Modeling soluble chemical transfer to runoff with rainfall impact as a diffusion process.Crossref | GoogleScholarGoogle Scholar |
Ahuja LR, Lehman OR (1983) The extent and nature of rainfall-soil interaction in the release of soluble chemicals to runoff. Journal of Environmental Quality 12, 34–40.
| The extent and nature of rainfall-soil interaction in the release of soluble chemicals to runoff.Crossref | GoogleScholarGoogle Scholar |
Baker JL (1980) Agricultural areas as nonpoint sources of pollution. In ‘Environmental impact of nonpoint source pollution’. (Eds MR Overcash, JM Davidson) pp. 275–310. (Ann Arbor Science Publishers Inc: Ann Arbor, MI)
Barnett AP, Carreker JR, Abruna F, Jackson WA, Dooley AE, Holladay JH (1972) Soil and nutrient losses in runoff with selected cropping treatments on tropical soils. Agronomy Journal 64, 391–395.
| Soil and nutrient losses in runoff with selected cropping treatments on tropical soils.Crossref | GoogleScholarGoogle Scholar |
Barrett JWH, Peterson SM, Batley GE (1991) The impact of pesticides on the riverine environment with specific reference to cotton growing. Report for the Cotton Research and Development Corporation and Land and Water Resources Research and Development Corporation. Cotton Research and Development Corporation and Land and Water Resources Research and Development Corporation.
Bates M (1993) Determination of the physico-chemical properties of HIH-2031 (DPX-PE350) according to EPA requirements; Report no. AMR 2506-92. EI duPont de Nemours & Co: DuPont Agricultural Products Experimental Station, Wilmington, Del., USA.
Bruns G, Tauber R (1992) Determination of KIH-2031 (DPX-PE350) in soil by Gas Chromatography/Mass Spectrometry (GC/MS). Report No AMR2421-92. EI duPont de Nemours & Co., DuPont Agricultural Products Experimental Station, Wilmington, Del., USA.
Connolly RD, Freebairn DM, Bridge BJ (1997a) Change in infiltration characteristics associated with cultivation history of soils in south-eastern Queensland. Australian Journal of Soil Research 35, 1341–1358.
| Change in infiltration characteristics associated with cultivation history of soils in south-eastern Queensland.Crossref | GoogleScholarGoogle Scholar |
Connolly RD, Kennedy IR, Silburn DM, Simpson BW, Freebairn DM (2001) Simulating endosulfan transport in runoff from cotton fields in Australia with the GLEAMS model. Journal of Environmental Quality 30, 702–713.
| Simulating endosulfan transport in runoff from cotton fields in Australia with the GLEAMS model.Crossref | GoogleScholarGoogle Scholar |
Cotham WE, Bidleman TF (1989) Degradation of malathion, endosulfan, and fenvalerate in seawater and seawater/sediment microcosms. Journal of Agricultural and Food Chemistry 37, 824–828.
| Degradation of malathion, endosulfan, and fenvalerate in seawater and seawater/sediment microcosms.Crossref | GoogleScholarGoogle Scholar |
Dang A, Silburn M, Craig I, Shaw M, Foley J (2016) Washoff of residual photosystem II herbicides from sugar cane trash under a rainfall simulator. Journal of Agricultural and Food Chemistry 64, 3967–3974.
| Washoff of residual photosystem II herbicides from sugar cane trash under a rainfall simulator.Crossref | GoogleScholarGoogle Scholar |
Edwards WM, Triplett GB, Kramer RM (1980) A watershed study of glyphosate transport in runoff. Journal of Environmental Quality 9, 661–665.
| A watershed study of glyphosate transport in runoff.Crossref | GoogleScholarGoogle Scholar |
Fillols E, Davis AM, Lewis SE, Ward A (2020) Combining weed efficacy, economics and environmental considerations for improved herbicide management in the Great Barrier Reef catchment area. Science of The Total Environment 720, 137481
| Combining weed efficacy, economics and environmental considerations for improved herbicide management in the Great Barrier Reef catchment area.Crossref | GoogleScholarGoogle Scholar |
Gerritse RG, Adeney JA, Sharma ML (1991) Henry’s law and the mobility of organochlorines in soils. In ‘Modelling the fate of chemicals in the environment’. (Ed. ID Moore) pp. 61–66. (CRES Australian National University: Canberra)
Glenn S, Angle JS (1987) Atrazine and simazine in runoff from conventional and no-till corn watersheds. Agriculture, Ecosystems & Environment 18, 273–280.
| Atrazine and simazine in runoff from conventional and no-till corn watersheds.Crossref | GoogleScholarGoogle Scholar |
Hornsby AG, Herner AE, Wauchope R (1996) ‘Pesticide properties in the environment.’ (Springer)
Hugo L (1999) Physico-chemical methods for containment of potential chemical contaminants on cotton farms. Unpublished PhD thesis, University of Sydney.
Isbell RF (2002) ‘The Australian soil classification.’ (CSIRO: Collingwood)
Isennsee AR, Sadeghi AM (1993) Impact of tillage practice on runoff and pesticide transport. Journal of Soil & Water Conservation 48, 523–527.
Leonard RA, Langdale GW, Fleming WG (1979) Herbicide runoff from upland Piedmont watersheds – data and implications for modeling pesticide transport. Journal of Environmental Quality 8, 223–229.
| Herbicide runoff from upland Piedmont watersheds – data and implications for modeling pesticide transport.Crossref | GoogleScholarGoogle Scholar |
Leonard RA, Knisel WG, Still DA (1987) GLEAMS: groundwater loading effects of agricultural management systems. Transactions of the ASAE 30, 1403–1418.
| GLEAMS: groundwater loading effects of agricultural management systems.Crossref | GoogleScholarGoogle Scholar |
Loch RJ, Foley JL (1994) Measurement of aggregate breakdown under rain – comparison with tests of water stability and relationships with field measurements of infiltration. Australian Journal of Soil Research 32, 701–720.
| Measurement of aggregate breakdown under rain – comparison with tests of water stability and relationships with field measurements of infiltration.Crossref | GoogleScholarGoogle Scholar |
Loch RJ, Robotham BG, Zeller L, Masterman N, Orange DN, Bridge BJ, Sheridan G, Bourke JJ (2001) A multi-purpose rainfall simulator for field infiltration and erosion studies. Australian Journal of Soil Research 39, 599–610.
| A multi-purpose rainfall simulator for field infiltration and erosion studies.Crossref | GoogleScholarGoogle Scholar |
Masters B, Rohde K, Gurner N, Reid D (2013) Reducing the risk of herbicide runoff in sugarcane farming through controlled traffic and early-banded application. Agriculture, Ecosystems & Environment 180, 29–39.
| Reducing the risk of herbicide runoff in sugarcane farming through controlled traffic and early-banded application.Crossref | GoogleScholarGoogle Scholar |
McDonald R, Baker D (1986) Soils of the left bank of the Nogoa River, Emerald irrigation area, Queensland. Agricultural Chemistry Branch Technical Report No. 15. Queensland Department of primary Industries, Brisbane.
Melland AR, Silburn DM, McHugh AD, Fillols E, Rojas-Ponce S, Baillie C, Lewis S (2016) Spot spraying reduces herbicide concentrations in runoff. Journal of Agricultural and Food Chemistry 64, 4009–4020.
| Spot spraying reduces herbicide concentrations in runoff.Crossref | GoogleScholarGoogle Scholar |
Peterson SM, Batley GE (1993) The fate of endosulfan in aquatic ecosystems. Environmental Pollution 82, 143–152.
| The fate of endosulfan in aquatic ecosystems.Crossref | GoogleScholarGoogle Scholar |
Powell B (1982) ‘Soils of the Gatton research station.’ Bulletin QB82005. (Queensland Department of Primary Industries: Brisbane)
Rayment GE, Higginson FR (1992) ‘Australian laboratory handbook of soil and water chemical methods.’ (Inkata Press: Melbourne, Australia)
Sharpley AN (1985) Depth of surface soil-runoff interaction as affected by rainfall, soil slope, and management. Soil Science Society of America Journal 49, 1010–1015.
| Depth of surface soil-runoff interaction as affected by rainfall, soil slope, and management.Crossref | GoogleScholarGoogle Scholar |
Sharpley AN, Smith SJ, Menzel RG (1982) Prediction of phosphorus losses in runoff from southern plains watersheds. Journal of Environmental Quality 11, 247–251.
| Prediction of phosphorus losses in runoff from southern plains watersheds.Crossref | GoogleScholarGoogle Scholar |
Silburn DM (2003) Characterising pesticide runoff from soil on cotton farms using a rainfall simulator. Unpublished PhD, University of Sydney, NSW, Australia.
Silburn DM, Connolly RD (1995) Distributed parameter hydrology model (ANSWERS) applied to a range of catchment scales using rainfall simulator data I: infiltration modelling and parameter measurement. Journal of Hydrology 172, 87–104.
| Distributed parameter hydrology model (ANSWERS) applied to a range of catchment scales using rainfall simulator data I: infiltration modelling and parameter measurement.Crossref | GoogleScholarGoogle Scholar |
Silburn DM, Glanville SF (2002) Management practices for control of runoff losses from cotton furrows under storm rainfall. I. Runoff and sediment on a black Vertosol. Australian Journal of Soil Research 40, 1–20.
| Management practices for control of runoff losses from cotton furrows under storm rainfall. I. Runoff and sediment on a black Vertosol.Crossref | GoogleScholarGoogle Scholar |
Silburn DM, Hunter HM (2009) Management practices for control of runoff losses from cotton furrows under storm rainfall. III. Cover and wheel traffic effects on nutrients (N and P) in runoff from a black Vertosol. Soil Research 47, 221–233.
| Management practices for control of runoff losses from cotton furrows under storm rainfall. III. Cover and wheel traffic effects on nutrients (N and P) in runoff from a black Vertosol.Crossref | GoogleScholarGoogle Scholar |
Silburn DM, Kennedy IR (2007) Rain simulation to estimate pesticide transport in runoff. ‘Rational environmental management of agrochemicals: risk assessment, monitoring and remedial action. ACS Symposium Series No. 966’. (Eds IR Soloman, KR Gee, SJ Crossan, AN Wang, S Sanchez-Bayo) pp. 120–135. (American Chemical Society) 10.1021/bk-2007-0966.ch008
Silburn DM, Simpson BW, Hargreaves PA (2002) Management practices for control of runoff losses from cotton furrows under storm rainfall. II. Transport of pesticides in runoff. Australian Journal of Soil Research 40, 21–44.
| Management practices for control of runoff losses from cotton furrows under storm rainfall. II. Transport of pesticides in runoff.Crossref | GoogleScholarGoogle Scholar |
Silburn DM, Foley JL, deVoil RC (2013) Managing runoff of herbicides under rainfall and furrow irrigation with wheel traffic and banded spraying. Agriculture, Ecosystems & Environment 180, 40–53.
| Managing runoff of herbicides under rainfall and furrow irrigation with wheel traffic and banded spraying.Crossref | GoogleScholarGoogle Scholar |
Silburn DM, Fillols E, Rojas-Ponce S, Lewis S, McHugh AD (2023) Direct comparison of runoff of residual and knockdown herbicides in sugarcane using a rainfall simulator finds large difference in runoff losses and toxicity relative to diuron. Science of The Total Environment 863, 160976
| Direct comparison of runoff of residual and knockdown herbicides in sugarcane using a rainfall simulator finds large difference in runoff losses and toxicity relative to diuron.Crossref | GoogleScholarGoogle Scholar |
Singh P, Jones RL (2002) Comparison of pesticide root zone model 3.12: leaching predictions with field data. Environmental Toxicology and Chemistry 21, 1552–1557.
| Comparison of pesticide root zone model 3.12: leaching predictions with field data.Crossref | GoogleScholarGoogle Scholar |
Snyder JK, Woolhiser DA (1985) Effects of infiltration on chemical transport into overland flow. Transactions of the ASAE 28, 1450–1457.
| Effects of infiltration on chemical transport into overland flow.Crossref | GoogleScholarGoogle Scholar |
Spencer W, Cliath M, Blair J, LeMast R (1985) Transport of pesticides from irrigated fields in surface runoff and tile drain water. USDA-ARS Conservation Research Report 31. US Government Printing Office, Washington DC.
Sumpter SR, Peterson BA, Ledeker KW, Mulderig LJ (1996) Analytical method for the determination of pyrithiobac sodium in soil using subcritical water extraction, graphitized carbon cleaned-up, and column-switching LC/UV analysis with confirmation by LC/MS. DuPont Report No AMR 2745-93. EI duPont.
Thorburn PJ, Wilkinson SN, Silburn DM (2013) Water quality in agricultural lands draining to the Great Barrier Reef: a review of causes, management and priorities. Agriculture, Ecosystems & Environment 180, 4–20.
| Water quality in agricultural lands draining to the Great Barrier Reef: a review of causes, management and priorities.Crossref | GoogleScholarGoogle Scholar |
Truman CC, Leonard RA, Davis FM (1998) GLEAMS-TC: a two-compartment model for simulating temperature and soil water content effects on pesticide losses. Soil Science 163, 362–373.
| GLEAMS-TC: a two-compartment model for simulating temperature and soil water content effects on pesticide losses.Crossref | GoogleScholarGoogle Scholar |
Wauchope RD (1992) Environmental risk assessment of pesticides: improving simulation model credibility. Weed Technology 6, 753–759.
| Environmental risk assessment of pesticides: improving simulation model credibility.Crossref | GoogleScholarGoogle Scholar |
Wauchope RD, Leonard RA (1980) Maximum pesticide concentrations in agricultural runoff: a semiempirical prediction formula. Journal of Environmental Quality 9, 665–672.
| Maximum pesticide concentrations in agricultural runoff: a semiempirical prediction formula.Crossref | GoogleScholarGoogle Scholar |
Weber JB (1972) Interaction of organic pesticides with particulate matter in aquatic and soil systems. In ‘Fate of organic pesticides in the aquatic environment’. Advances in Chemistry Series 111’. (Ed. R Gould) pp. 55–120. (American Chemical Society: Washington)
Willis GH, McDowell LL, Murphree CE, Southwick LM, Smith S (1983) Pesticide concentrations and yields in runoff from silty soils in the lower Mississippi valley. Journal of Agricultural and Food Chemistry 31, 1171–1177.
| Pesticide concentrations and yields in runoff from silty soils in the lower Mississippi valley.Crossref | GoogleScholarGoogle Scholar |
Wockner G, Freebairn DM (1991) Water balance and erosion study on the Eastern Darling Downs – an update. Australian Journal of Soil and Water Conservation 4, 41–47.
Young DF, Fry MM (2019) Field-scale evaluation of pesticide uptake into runoff using a mixing cell and a non-uniform uptake model. Environmental Modelling & Software 122, 104055
| Field-scale evaluation of pesticide uptake into runoff using a mixing cell and a non-uniform uptake model.Crossref | GoogleScholarGoogle Scholar |
1 ‘Sediment concentration’ sediment mass per litre of water. In contrast, pesticide concentration in sediment is referred to as ‘diuron concentration in sediment’ (mg kg−1).
2 Determined from regression analysis of ERO against DSS. ERO ranged from 37.1 (trifluralin) to 16 (α-endosulfan) but ANOVA was not useful in revealing differences between pesticides, because ERO decreased with time since spraying and increasing the variance, leading to an l.s.d. of 10. When trifluralin was excluded, differences in ERO between pesticides were not significant.


