A model of daily mean canopy conductance for calculating transpiration of olive canopies
Francisco Orgaz A , Francisco J. Villalobos A B , Luca Testi A C and Elias Fereres A BA Instituto de Agricultura Sostenible, CSIC, Alameda del Obispo, S/N, 14004 Córdoba, Spain.
B Departamento de Agronomia, Universidad de Córdoba, Apartado 3048, 14080 Córdoba, Spain.
C Corresponding author. Email: ag2lucat@uco.es
Functional Plant Biology 34(3) 178-188 https://doi.org/10.1071/FP06306
Submitted: 16 November 2006 Accepted: 31 January 2007 Published: 22 March 2007
Abstract
We tested the hypothesis that the transpiration (λEp) of high-coupled canopies, such as olive groves, may be calculated on a daily basis with sufficient precision by the Penman–Monteith ‘big leaf’ equation, by a model of bulk daily canopy conductance (gc) capable of scaling for canopy dimension. Given the limited data required, such a model could replace the standard approach (ET0 × Kc) for calculating olive water requirements, enhancing the precision of estimates. We developed a specific model of daily gc for unstressed olive canopies that was calibrated by transpiration measurements obtained by water balance from a 2-year experiment in a mature orchard with λEp ranging from 0.6 (February 1993) to 11.5 (July 1994) MJ m–2 day–1 and where leaf area index (L) changed from 1.25 to 2.5. The model uses the intercepted fraction of daily PAR and a linear function of average daytime temperature. The model was validated with λEp data collected by eddy covariance in a 3-year experiment conducted in a growing orchard that differed in L and cultivar from the one used in the calibration. The gc model, when used in the Penman–Monteith equation, gave very good daily λEp predictions for all seasons during 3 years, ranging from 0.5 (November 1998) to 5.5 (June 2000) MJ m–2 day–1, indicating that the goals of dealing with the dependence of olive gc on L and of simulating the seasonal variations in gc were achieved. A comparison with the Jarvis gc model, calibrated with 2 months of measured gc hourly data, showed that the gc model developed here performed better than the Jarvis model for the 3-year dataset. The exception to this was the period in which the Jarvis model was calibrated. This indicates that (1) the Jarvis model did not account for the seasonal variations in gc of the olive trees; and (2) the spatial and temporal scale assumptions required in the calibration of gc generate seasonal errors in the simulated bulk daily λEp for this crop. The applicability of this bulk gc model is restricted to well watered olive canopies and to the one-layer approach of calculating λEp but it could be adapted to rain-fed canopies in the future.
Additional keywords: olive tree, Penman–Monteith equation, stomatal conductance.
Anderson MC,
Norman JM,
Meyers TP, Diak GR
(2000) An analytical model for estimating canopy transpiration and carbon assimilation fluxes based on canopy light-use efficiency. Agricultural and Forest Meteorology 101, 265–289.
| Crossref | GoogleScholarGoogle Scholar |
and 17, depending on the cloudiness. The model determines the actual cloudiness of the day by the atmospheric transmissivity (τatm, non-dimensional), defined as the ratio between the actual (Rs, input) and the extraterrestrial (Rext, function of day of year and latitude) solar radiation. The value of the fraction of intercepted PAR radiation is then found by interpolation, assuming τatm = 0.8 and Q = Qdtot in clear sky conditions and τatm = 0.2 and Q = Qdd in completely overcast days. This simplified model is olive-specific in the presented parameterisation, but can be adapted for other tree crops if PAR interception data are available.
Appendix 2: Jarvis-type stomatal model
We used the well known multiplicative stomatal conductance model of (Jarvis 1976) as a basis for comparison with our model. The Jarvis model was applied (hourly) to the data of Experiment 2, in the form described by Dolman et al. (1988), but without the soil moisture deficit function (our trees were irrigated). The original function of L was replaced with an empirical function calibrated specifically.

The individual functions are:
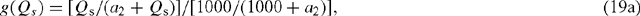



where


and where Qs, incoming solar radiation (W m−2), δq, specific humidity deficit (g kg−1), T, air temperature (°C) and L, leaf area index. The values of the fitted parameters a1 − a5 are given in Table 1; TL and TH are taken as 0 and 40°C, respectively.


