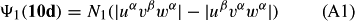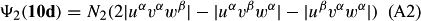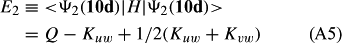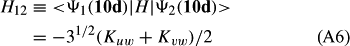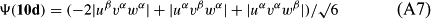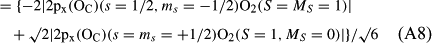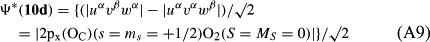Valence Bond Formulations of Mechanisms for the Formation and Decomposition of N2O5

Richard D. Harcourt; Thomas M. Klapötke

doi:10.1071/EN06058

RESEARCH ARTICLE

Previous Next Contents Vol 3(5)

Valence Bond Formulations of Mechanisms for the Formation and Decomposition of N₂O₅

Richard D. Harcourt ^A ^C and Thomas M. Klapötke ^B

+ Author Affiliations

- Author Affiliations

^A School of Chemistry, University of Melbourne, Parkville, Vic. 3010, Australia.

^B Department of Chemistry and Biochemistry, Ludwig-Maximilian University Munich (LMU), Munich D-81377, Germany.

^C Corresponding author. Email: r.harcourt@unimelb.edu.au

Environmental Chemistry 3(5) 355-363 https://doi.org/10.1071/EN06058
Submitted: 4 September 2006 Accepted: 9 October 2006 Published: 26 October

Environmental Context. N₂O₅ is an important nitrogen reservoir in polar stratospheric clouds found in Antarctica and involved with the ozone hole. Here we provide valence bond representations for the gas-phase formation and decomposition of this molecule.

Abstract. Qualitative valence bond considerations are used to suggest how electronic reorganization could proceed for (a) the formation of N₂O₅ via the reactions NO₂ + O₃ → NO₃ + O₂, and NO₂ + NO₃ → N₂O₅, and (b) the thermal decomposition of N₂O₅ via the following sets of reactions: (i) N₂O₅ → NO₂ + NO₃, 2NO₃ → O₂NOONO₂ →2NO₂ + O₂; (ii) NO₂ + NO₃ → ONOONO₂ → NO + O₂ + NO₂, NO + NO₃ → 2NO₂. Increased-valence structures, which possess one-electron bonds and fractional electron-pair bonds as well as ‘normal’ electron-pair bonds, are used to represent the electronic structures of the molecules.

Keywords. : atmospheric chemistry — nitrogen oxides — ozone — valence bond structures

Acknowledgements

T.M.K. gratefully acknowledges financial support from the University of Munich (LMU), the Fonds der Chemischen Industrie and the European Research Office (ERO) of the USA Army Research Laboratory (ARL) under contract no. N 62558–05-C-0027. We thank Mrs. Carmen Nowak, at the LMU, for drawing the VB structures, and Professor Brian Duke for reading the paper and for his helpful comments. R.D.H. also thanks Dr Jonathan White for the provision of a workstation.

References

Appendix: Electronic States for the O₂ of X + O₃ → XO + O₂

In ref. [10a], it has been deduced that X + O₃ → XO + O₂ generates O₂(³Σ^–_g). We shall re-describe the theory here, using real rather than the complex MOs of ref. [10a] for the O₂. To describe the O₂–NO₃ dissociation of the O_AO_B–O_CNO₂ product-like structure 10d of Fig. 10, the primary active space involves three electrons, which singly occupy the 2p_x(O_C) AO, the π*_x(O_AO_B) MO and the π*_y(O_AO_B) MO. We shall designate these (assumed normalized) orbitals as u, v and w, respectively. Well before the conclusion of the reaction, the u and v orbitals overlap, to form the fractional O_AO_B–O_C bond in 10d when their electrons are singlet spin-paired. The resulting S = M_S = 1/2 spin wave function for the u, v and w electrons is given by Eqn (A1).

A second, orthogonal S = M_S = 1/2 spin wave function for the three electrons, is that of Eqn (A2).

At any stage along the reaction coordinate, the wave function of Eqn (A3)

can be constructed. Near the conclusion of the reaction, the u–v overlap is negligible, and therefore the orbital overlap integral < u|v > can be ignored. The resulting values for the normalization constants of Eqns (A1) and (A2) are then N₁ = 1/√2 and N₂ = 1/√6. We can then construct the energy matrix elements of Eqns (A4)–(A6).

in which Q is the energy of three Slater determinants without exchange (i.e. with no antisymmetry), and the K_ij = < i(1)j(2)|1/r₁₂|j(1)i(2) > are exchange integrals for pairs of the u, v and w orbitals. When dissociation of the O_AO_B–O_CNO₂ bond occurs, the exchange integrals K_uv and K_uw are equal to zero, to give E₁ = Q – 1/2K_vw, E₂ = Q + 1/2K_vw and H₁₂ = –3^1/2K_vw/2. Using these matrix elements, the lower-energy solution of the associated secular equation gives E = Q - K_vw, C₁ = 3^1/2/2 and C₂ = 1/2. The resulting expression for the Ψ(10d) of Eqn (A3) is that of Eqn (A7),

which is equivalent to Eqn (A8). The O₂ (as O_AO_B) component of Eqn (A8) involves the O₂(³Σ^–_g) ground-state with M_S = +1 and 0 spin components.

The higher-energy solution of the secular equation gives E* = Q + K_vw, C₁ = 1/2, C₂ = –3^1/2/2 and Eqn (A9)

The O₂ component of Eqn (A9) involves the O₂(¹Δ_g) excited-state.

Valence Bond Formulations of Mechanisms for the Formation and Decomposition of N2O5

Acknowledgements

Appendix: Electronic States for the O2 of X + O3 → XO + O2

Subscriber Login

Valence Bond Formulations of Mechanisms for the Formation and Decomposition of N₂O₅

Appendix: Electronic States for the O₂ of X + O₃ → XO + O₂