Recounting bias can affect abundance estimates from intensive helicopter surveys of feral goats
John P. Tracey A B and Peter J. S. Fleming A C D E *
A C D E *
A Institute of Applied Ecology, University of Canberra, 11 Kirinari Street, Bruce, ACT 2617, Australia.
B Biosecurity and Food Safety, New South Wales Department of Primary Industries, Prince Street, Orange, NSW 2800, Australia.
C Vertebrate Pest Research Unit, New South Wales Department of Primary Industries, Orange Agricultural Institute, 1447 Forest Road, Orange, NSW 2800, Australia.
D School of Environmental and Rural Science, University of New England, Armidale, NSW 2351, Australia.
E Centre for Sustainable Agricultural Systems, University of Southern Queensland, Toowoomba, Qld 4350, Australia.
Wildlife Research 50(5) 389-397 https://doi.org/10.1071/WR22097
Submitted: 8 June 2022 Accepted: 12 October 2022 Published: 11 November 2022
© 2023 The Author(s) (or their employer(s)). Published by CSIRO Publishing. This is an open access article distributed under the Creative Commons Attribution-NonCommercial-NoDerivatives 4.0 International License (CC BY-NC-ND)
Abstract
Context: Aerial surveys are widely used for estimating the abundance of wildlife over large areas. The failure to count all animals within survey transects is commonly acknowledged and there are many techniques to measure and correct for underestimation. However, the possibility of animals being counted more than once in intensive surveys, which leads to overestimation, is rarely examined. Animals can move in response to observers or vehicles, and bias can occur when animals move before or after detection. Movement of animals immediately prior to and associated with observation processes is methodologically accommodated in distance sampling but bias attributable to responsive movement after observation platforms have passed requires investigation.
Aims: We sought to investigate potential biases caused by animal movement during intensive helicopter surveys of feral goats, and to quantify the probability that animals are available for recounting because of their responsive movements.
Methods: Using ground-based behavioural studies simultaneous with intensive helicopter strip surveys of feral goats, we measured the extent of responsive movement, distances and directions moved, and sampling design parameters, and contrasted those with random movements.
Key results: Feral goats did not move randomly in response to helicopters. Animals within the transect strips, and therefore potentially visible from the aircraft, were more likely to move than those outside the transect. Considerable responsive movement (flushing) occurred between transects and more animals (64%, n = 448) moved towards unsampled transects than towards transects already sampled. Because of the spatial separation of transects, 21% of goats were available for recounting in adjacent transects, leading to potential overestimation.
Conclusions: Although most extensive surveys of macropods and other wildlife in Australia account for overestimation in their design, surveys that sample intensively and apply valid corrections for undercounting are likely to produce positively biased estimates of abundance where flushing occurs. Likewise, intensive thermal surveys could be subject to positive bias for animals prone to flushing. This is routinely ignored in wildlife management and research where close transects are used to estimate abundance.
Implications: Responsive movement requires consideration when designing intensive aerial surveys of wildlife. Randomised transects without replacement or larger distances between transects will counteract recounting bias.
Keywords: aerial survey, antipredator response, Capra hircus, density, feral goat, responsive movement, ungulate, wildlife management.
Introduction
Estimating population variables is a fundamental process for wildlife management and the methods used affect accuracy, precision, reliability and utility of ecological knowledge (Elton 1927; Elphick 2008; Fryxell et al. 2014). Strip and delimited and unbounded transect surveys are commonly used to aerially estimate wildlife abundance and each methodology and analysis depends on various assumptions. Transect surveys have used distance sampling (van Hensbergen et al. 1996; Walter and Hone 2003; Buckland et al. 2004), multiple observers (Rice and Harder 1977; Caughley and Grice 1982; Tracey et al. 2005; Melville et al. 2008), radio-tagging (e.g. Rice and Harder 1977; Packard et al. 1985; Ries et al. 1998), regression techniques (e.g. Caughley et al. 1976; Hone 1986), indices (e.g. Tracey et al. 2005), and comparison with independent population estimates (Short and Hone 1988; Jachmann 2002; Greene et al. 2017).
When sampling without replacement, most estimators implicitly or explicitly assume that animals are not counted more than once, nor consistently missed (e.g. distance sampling, Buckland et al. 2004), or that they are detected prior to any movement in response to the observer. Line-transect and strip-transect sampling are instantaneous methods that assume that objects are in a fixed location at the time of the survey, are all detected on the transect line and are detected at their initial location (Buckland et al. 2001, 2004; Fewster et al. 2005). Whereas plants and inanimate objects are stationary (e.g. beans, Hone 1986), animals move at various rates and directions, which instils potential responsive movement biases for animal surveys. In most transect surveys, observers or their observation platform will cause animals to move, particularly walked line transects (e.g. Southwell 1994) and low-level aerial surveys (e.g. Fleming and Tracey 2008).
In distance sampling and strip-transect sampling, movements prior to detection have been considered and accommodated (Buckland et al. 2004; Fewster et al. 2008). Borchers et al. (1998) used a capture–recapture method from two survey platforms to correct for both the failure to observe animals on the line and the effects of responsive movement. Palka and Hammond (2001) estimated the distance at which responsive movement commenced by using animal orientation, then applied a capture–recapture method separately for observations within (‘close’) and beyond (‘far’) this distance.
Responsive movement bias is assumed to be small if animals move at random and if the animal movement is slow relative to the speed of the observer (Buckland et al. 2001; Glennie et al. 2015). Most estimators will not be adversely affected if animals have an equal chance of moving into or out of a transect. However, bias can occur when animals consistently move away from observers (Palka and Hammond 2001; Linklater and Cameron 2002; Tracey and Fleming 2007) or towards observers (Palka and Hammond 2001; Cañadas et al. 2004).
Aerial surveys of wildlife are usually designed by using standard random (e.g. Choquenot 1995) or systematic (e.g. Walter and Hone 2003) sampling procedures. Systematic designs with a random start usually provide more robust estimates and are easier to implement in the field than are designs where transects are placed randomly without replacement (Buckland et al. 2015) A common practice is to saturate the study area with a grid of transects and select a subset randomly without replacement (Choquenot 1995; Pople et al. 1998a; Fleming et al. 2000). However, in both designs, transects are typically flown consecutively from one edge of the study site to the other to reduce ferry time and operational costs (e.g. Tracey et al. 2005). In such situations, assumptions are likely to be violated by individuals flushing unidirectionally between transects.
We could find only one reference relating to animal movements into other sampled areas after detection or the passage of observers or observation platforms (Terletzky and Koons (2016) found 4.4% mean duplicate observations of GPS-collared bison, Bison bison, aerially surveyed with topography-following flight paths), and none investigating potential positive survey bias or cumulative bias with subsequent passes. Here, we sought to investigate the possibility of recounting bias in helicopter counts of feral goats (Capra hircus) and used independent ground observations to quantify any such biases.
Materials and methods
Study site
The study site with a high density of feral goats was chosen near the Coolah Tops National Park (−32°0′S, 149°58′E) in central-eastern New South Wales, Australia. The region has high rainfall (739 mm average annual rainfall, s.e. = 23.2, n = 55 years) and medium to high elevation (620–1190 m). The topography is rugged, encompassing gorges, narrow creeklines, wide valley floors, undulating to steep hillsides, narrow ridge tops and broad plateaux. Vegetation communities ranging from open grasslands to tall forests are supported by the fertile soils derived from basalt (Banks 1998). The overstorey associations are mainly woodlands of Eucalyptus goniocalyx, E. melliodora, E. viminalis, and E. albens, and gallery forests of Casuarina cunninghamiana are interspersed. High densities of feral goats, eastern grey kangaroos (Macropus giganteus), common wallaroos (Osphranta robustus), Australian merino sheep (Ovis aries) and cattle (Bos taurus) occurred at the site.
Aerial surveys
Methods follow those described in Tracey et al. (2005) and Tracey and Fleming (2007). Between 1997 and 2002, 34 surveys were flown over the study area in either a Hughes 500 or Bell Jetranger (206B) helicopter. During surveys, the helicopter maintained an approximately constant height of 150 ft (~46 m) above ground level (agl) and a constant airspeed of 45 knots (~85 km h−1). Strip transects of 100 m on each side were delimited by right-angled poles attached to the helicopter and transects were spaced 300 m apart. Doors were removed and observers, one behind the pilot and two on the passenger (double) side, looked to the side of the helicopter and forward vision was obscured for consistency of search area on the double-observer side. Subsequently, there was a blind strip of 60 m below the helicopter where animals could not be observed by on-board observers. Transects (6–14 km) were selected at random without replacement and oriented east–west, which was perpendicular to the orientation of the major valley systems, and first passes progressed from north to south. No adjacent transects were flown consecutively, so randomly selected transects that were consequently omitted on first pass were subsequently flown in a second pass from north to south. The whole site was flown in one session for each survey. Surveys were flown within 3 h of either sunrise or sunset, and each survey was sampled between 40% and 70% of the site area.
Ground observations
During aerial surveys, 6–12 experienced ground-based observers located, counted and monitored behaviour and movements of free-ranging goats before, during and after helicopter passes. To reduce disturbance, the ground observers, assisted by radio-telemetry, spotted herds of goats from high vantage points and approached them on foot behind cover and as quietly as possible. Observations were not conducted on the rare occasions that goats were noticeably affected by ground observers, that is, where goats exhibited any alert response to the presence of an observer. Independent behaviour data suggest that fewer than 7% of observed groups were disturbed by observers (n = 22 184, P. Fleming, unpubl. data). Because ground observers could not predict whether a particular group of goats would occur within transect areas simultaneously with survey overflights, many observations of feral goat groups were obtained (>1500), but we could use only 784 in analyses. Although macropods were aerially counted at the same time as goats, lack of local knowledge of macropod dispersion throughout the site and limited available personnel precluded deployment of observers to take simultaneous ground observations of macropods.
Observers recorded date, time and site of the observation, and the number, colour, sex and age ratio, location, activity, vegetation type, and movements of goat groups, and other variables. Horizontal distances to the helicopter from observed goats were also recorded using line-of-sight, 1:25 000 topographic maps, grid locations and a global positioning system (GPS). Prior to the helicopter being heard or becoming visible to the observer, grid locations of animals were mapped, their activity recorded, and horizontal distances were subsequently estimated with simultaneous helicopter locations that were calculated from its on-board GPS. All observations were conducted during aerial surveys and helicopters used radar altimeters to maintain a fixed height and therefore the recorded horizontal distance was directly proportional to the actual distance between the observed animals and the helicopter. When individual feral goats within a group behaved differently, the activity of most animals in the group was recorded.
Probability that animals are available for recounting
Here we estimated the probability that animals once available for detection in a sampling area were available again in adjacent sample areas. This probability relies on information about the extent of responsive movement, the direction of movement, the distances between transects and the sampling design (sample intensity, transect width, distance between transects, and distance unobservable beneath the aircraft).
The probability that an animal or group is available for recounting (pa) was estimated using the following equation:

where pmi is the probability an animal moves to i other transects; psi is the probability Transect i is sampled; and the maximum value for i is the maximum perpendicular distance moved divided by the distance between transects.
The probability of an animal moving into another transect (pmi) is dependent on the transect width, the distance between the transects, the proportion of animals that move, how far they travel (dm) and in which direction they travel (θm). These parameters were observed or later estimated for feral goats known from observations to be within sampled transects during surveys. We converted dm and θm to the perpendicular distance moved in relation to east–west transects (dp, Fig. 1).
We selected a Weibull distribution  for describing the relationship between the probability of goats moving into another transect (y = pmi) and the perpendicular distance moved (x = dp; Evans et al. 1993). This relationship was selected over the simple exponential function because its shape could be altered according to the γ-value. Scale (λ) and shape (γ) parameters were optimised using maximum log-likelihood estimation, assuming a multinomial error structure.
for describing the relationship between the probability of goats moving into another transect (y = pmi) and the perpendicular distance moved (x = dp; Evans et al. 1993). This relationship was selected over the simple exponential function because its shape could be altered according to the γ-value. Scale (λ) and shape (γ) parameters were optimised using maximum log-likelihood estimation, assuming a multinomial error structure.
The probability of sampling a transect depends on survey design and the initial sampling rate. If parallel transects are sampled consecutively from one edge of the study site to the other, as is common practice (e.g. Reilly et al. 2017), only animals that move in one direction are able to be recounted. However, if multiple passes are made, or transects are sampled in a random order, the calculations of pmi need to be adjusted, as animals that move in either direction may be available during subsequent passes.
Therefore, the sampling probability for two passes can be estimated by calculating separate probabilities for the first and second pass, where:

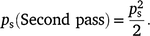
On the second pass, goats that moved in either direction may be available for sampling, hence
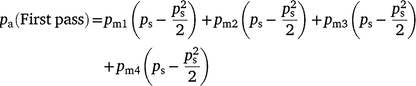

The Weibull function was used to estimate the probability that a group moved into another transect in relation to the transect spacing, i.e.
-
east–west transects were placed 300 m apart,
-
100 m was sampled on either side of the helicopter,
-
60 m was unavailable directly underneath the helicopter (i.e. 30 m each side of the centre line), and
-
because of 1 and 2, 40 m was unsampled between transects.
Results
Feral goat movement patterns
Goats were significantly more likely to move south if they were initially south of the helicopter (χ2 = 16.6, d.f. = 1, P < 0.001) and more likely to move north when they were initially north of the helicopter (χ2 = 16.8, d.f. = 1, P < 0.001). Combining these ratios, 64% of individual goats moved away from the helicopter and 36% moved towards it (n = 448).
Probability that animals are available for recounting
For groups of goats that occurred within sampled transects, the probability of moving, pm, decreased with the perpendicular distance travelled, dp (Fig. 2,  ). Over 60% of groups moved, but only 30% moved further than 100 m and 8% further than 400 m.
). Over 60% of groups moved, but only 30% moved further than 100 m and 8% further than 400 m.
|
|
Because consecutive transects were omitted on the first pass, the probability of sampling an adjacent transect was 0 and probabilities of moving into more than one transect were calculated only for those animals that moved in one direction. Because the probability of goats moving was ≠1 when the perpendicular distance = 0, the Weibull function was adjusted using the observed proportion of goats moving when the helicopter was directly overhead (i.e. 0.61). The maximum recorded perpendicular distance moved was 1200 m (mean = 104 m, median = 12 m). The probabilities of goats moving (pm) perpendicular to the helicopter were estimated using the proportion of goat groups observed; hence, these calculations assumed that the distance moved was not a function of group size (Tracey and Fleming 2007).
As goats moved unevenly in southern and northern directions relative to the helicopter, probabilities were estimated separately for each side and were weighted for direction moved according to the observed proportions (i.e. 0.64 ‘away’ and 0.36 ‘towards’). The probability of a group moving into another transect was estimated by averaging probabilities predicted for perpendicular distance moved (Fig. 2). These predicted probabilities were calculated separately for the range of distances to each adjacent transect (Table 1).
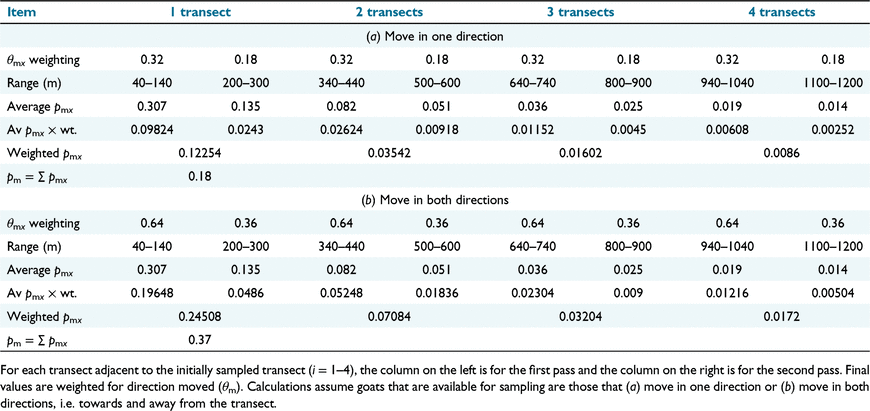
|
Assuming that transects are flown consecutively from one edge of the study site to the other, as is usually the case, the probability of animals moving into an adjacent transect occurs in only one direction. For example, if transects are flown from north to south, goats that move north will not be available in another transect. Hence the probability of animals moving south is 0.18 for goats on the northern side (1/2 of 0.36) and 0.32 for goats on the southern side (1/2 of 0.64).
We sampled all available transects and so the probability of sampling an adjacent transect (ps) was 1. Despite sampling all transects, 100 m of every 300 m transect was unavailable (60 m underneath plus 40 m between transects), which translates to an achieved sampling rate of 67%. Using the pa and pm values (Table 1), and assuming that transects were sampled consecutively, the probability that a group of goats was available for recounting (pa) would be 0.18. When estimating seasonal abundance for the whole site, we sampled 18 of 28 possible transects. Hence, the probability of sampling a transect (ps) was 0.64 (=18/28), which translates to a sampling rate of 43% when considering the area unavailable under the aircraft and between transects. In this case, the probability that goats were available for recounting (pa) was 0.12 (=0.64 × 0.18).
However, because consecutive transects were omitted on the first pass and sampled on a second pass, probabilities were calculated separately.

where pm is calculated using Table 1a, ps = 1, ps (First pass) = 0.5

where pm is calculated using Table 1b, ps = 1, ps (Second pass) = 0.5

Discussion
The failure to count all animals within a defined sample area or distance classes has dominated discussions about limitations of aerial survey methods, and correction for underestimation is ubiquitous (e.g. Caughley et al. 1976; Barker 2008; Laake et al. 2008). Even when duplicate counting has been identified, estimates were still inflated to account for overall under-detection (Terletzky and Koons 2016). However, we have shown overestimation as a potential problem that needs to be designed for in aerial surveys. Although the potential for recounting animals has often been assumed to be negligible (Seber 1982; Beasom et al. 1986; Linklater and Cameron 2002) or not considered because of small movements (e.g. Lethbridge et al. 2019), our results showed that under intensive sampling, movement between transects can be substantial (here 18–21%, depending on sampling rate) and, consequently, can cause large overestimates of density and abundance.
Assuming that animal movement is independent of the observer, bias from that movement is related to the distance animals travel during survey passage (Glennie et al. 2015). Negative bias in line-transect and strip sampling is smaller when mean animal speed is less than observer speed and larger when animal speed is greater than observer speed (Glennie et al. 2015). However, animal movement is not independent of observers and flight responses to observers and observer platforms are common (Clancy et al. 1997; Stankowich 2008), particularly among large herbivorous prey animals such as ungulates and macropods (e.g. Krausman et al. 1986; Pople et al. 1998b; Grigg et al. 1999; Tracey and Fleming 2007). We also showed that the direction of feral goat movements was not uniform.
The distance ungulates travel in response to aerial disturbance, and hence the potential for recounting, is difficult to compare across studies because elevation, aircraft speed, distance to the aircraft and type of aerial activity are widely varied and, in some cases, not reported. During very low-flying helicopter surveys (10–15 m agl), Beasom et al. (1986) reported resightings of marked white-tailed deer (Odocoileus virginianus) between 0% and 13% of total deer seen. Intensive sampling resulted in multiple sightings of individual deer, but it was concluded that this rendered total counts less conservative (Beasom et al. 1986). However, the proportion of marked deer in the population was not reported, and only 26–40% of deer were observed. Hence, the reported figure of 13% for a 100% sampling intensity would indicate that a higher proportion was actually available for recounting (i.e. between 33% and 50%). The low altitudes flown (e.g. DeYoung et al. 1989) and the recorded flushing behaviour of ungulates in other studies (e.g. Linklater and Cameron 2002; Stankowich 2008; Glennie et al. 2015) would also suggest greater movement between transects than has been reported.
Our probability of recounting was negatively correlated with sampling rate and positively correlated with the distance feral goats moved between transects. Both correlations are likely to hold true for any intensive aerial transect survey of large mobile animals involving species with a fleeing antipredator response. Despite some species- and situation-specific flushing responses, recounting is highly unlikely in aerial surveys that have widely spaced transects (e.g. macropod surveys; Cairns et al. 1991; Pople et al. 2006; Lunney et al. 2018), and undercounting persists in those cases. However, studies that sample intensively to achieve higher accuracy in low-density populations or localised high-density populations (e.g. Melville et al. 2008; Reilly et al. 2017), and apply valid corrections for an inability to detect all animals are likely to produce positively biased estimates of density. Recounting is more likely for intensive or low-level surveys of large ungulates that flush large distances, such as, for example, caribou (Rangifer tarandus; Calef et al. 1976; Harrington and Veitch 1991), mule deer (Odocoileus hemionus; Krausman et al. 1986), and mountain goats (Oreamnos americanus; Côté 1996). Linklater and Cameron (2002) found that recounting of feral horses (Equus caballus) resulted in overestimates of 15–32% in the Kaimanawa Ranges of New Zealand. Their Hughes 500 helicopter was flown at 60 m agl and horses were reported moving 0.1–2.75 km in response to the helicopter, but their sampling intensity was maximised to achieve a census.
All the above ungulate species and feral goats are gregarious, and, although detectability is associated with groups size (e.g. Samuel and Pollock 1981; Melville et al. 2008; Tracey et al. 2008), it is possible that flight responses to human disturbance are greater in larger groups (Stankowich 2008). Unmanned aerial vehicles (UAVs) cause greater flight distances in larger groups of guanacos (Lama guanicoe) than in small groups or solitary animals (Schroeder and Panebianco 2021). Other solitary larger herbivores, including ungulates such as the Japanese serow (Capricornis crispus), might be less likely to flush great distances in response to human disturbance, particularly once cover is reached (Takada et al. 2019). Different social organisation and antipredator responses in ungulates (Jarman 1974) and macropods (Jarman 1991) might affect flushing behaviour and probability of recounting during aerial surveys, but further study is required to elucidate and quantify resultant estimation biases.
If transects are close relative to the distance that an animal can traverse in responsive movement, then the measurements of interest are the speed of the animal and the observer between successive transects, and the direction of movement. The threshold below which feral goats are more likely to flush is 150 m from the helicopter (Tracey and Fleming 2007), which is within the observable range of observers and close to the outer-strip boundary for transects sampled in our study. If the helicopter was within this distance, goats travelled further (>150–2500 m) from the point of disturbance, which has implications for the probability that animals are available for recounting. We concluded that goats did not have an equal chance of moving into or out of a transect, because those that were potentially visible moved significantly further than those that were outside the transect area. Full randomisation of transect placement, without replacement, has potential to reduce the possibility of directional responsive movement or to increase the likelihood that movements into and out of subsequent transects are homogenous: there is software available to facilitate logistically efficient random placement of transects (e.g. Marshall 2019). However, because flushing is often in response to the noise of low-level aerial survey platforms, including drones (e.g. Brunton et al. 2019), there is an increased likelihood of animals moving away from surveyed areas where transect lines randomly fall close together, thereby interfering with the assumptions of fixed location and sighting at initial locations.
Most of the few studies comparing aerial survey estimates with actual numbers confirm underestimation (Caughley 1974), for a review; e.g. Tracey et al. 2008), which suggests that recounting animals is uncommon and that conservative estimates of density prevail (e.g. Beasom et al. 1986; Lunney et al. 2018). However, as technologies, such as surveys using thermal imagery (e.g. Havens and Sharp 1998; Cox et al. 2021) and videography (e.g. Catling and Coops 2004), UAV-assisted thermal imagery surveys (e.g. Lhoest et al. 2015; Witt et al. 2020) and estimation procedures improve, elimination of recounting is of increasing importance to ensure that estimates are not positively biased, which is especially relevant under high-intensity sampling regimes.
Data availability
Data are available from the authors on request.
Conflicts of interest
The authors declare no conflicts of interest.
Declaration of funding
The Australian Government’s National Feral Animal Control Program – Natural Heritage Trust, and Wildlife and Exotic Disease Preparedness Program, and New South Wales Department of Primary Industries funded the research.
Acknowledgements
Mike, Ant and Chris Martin, Doug and the late Donald Arnott, and Kevin Cluff kindly provided access to their land where the surveys were conducted. Greg Jones, Matthew Gentle, Ryan Breen, Brian Lukins, Ken England, Glen Walker and Richard Mason assisted with the main field work and Matt Hollingdale and Mark Rogers flew all the surveys. This work was supervised by Jim Hone and Glen Saunders and is adapted from JPT’s MAppSc thesis: Tracey JP (2004) Assessing estimators of feral goat (Capra hircus) abundance. (University of Canberra: Belconnen). Bernie Dominiak edited the paper, which also benefitted from the critiques of Dan Parker and an anonymous referee. The project was approved by the Orange Animal Ethics Committee, Approval ORA 97/004.
References
Banks RG (1998) Soil landscapes of the Blackville 1:100,000 sheet report. (Department of Land and Water Conservation: Sydney)Barker, R (2008). Theory and application of mark–recapture and related techniques to aerial surveys of wildlife. Wildlife Research 35, 268–274.
| Theory and application of mark–recapture and related techniques to aerial surveys of wildlife.Crossref | GoogleScholarGoogle Scholar |
Beasom, SL, Leon, FG, and Synatzske, DR (1986). Accuracy and precision of counting white-tailed deer with helicopters at different sampling intensities. Wildlife Society Bulletin 14, 364–368.
Borchers, DL, Buckland, ST, Goedhart, PW, Clarke, ED, and Hedley, SL (1998). Horvitz-Thompson estimators for double-platform line transect surveys. Biometrics 54, 1221–1237.
| Horvitz-Thompson estimators for double-platform line transect surveys.Crossref | GoogleScholarGoogle Scholar |
Brunton, E, Bolin, J, Leon, J, and Burnett, S (2019). Fright or flight? Behavioural responses of kangaroos to drone-based monitoring. Drones 3, 41.
| Fright or flight? Behavioural responses of kangaroos to drone-based monitoring.Crossref | GoogleScholarGoogle Scholar |
Buckland ST, Anderson DR, Burnham KP, Laake JL, Borchers DL, Thomas L (2001) ‘Introduction to distance sampling: estimating abundance of biological populations.’ (Oxford University Press: Oxford)
Buckland ST, Anderson DR, Burnham KP, Laake JL, Borchers DL, Thomas L (2004) ‘Advanced distance sampling.’ (Oxford University Press: Oxford)
Buckland ST, Rexstad EA, Marques TA, Oedekoven CS (2015) ‘Distance sampling: methods and applications.’ (Springer-Verlag)
Cairns, SC, Pople, AR, and Grigg, GC (1991). Density distributions and habitat associations of red kangaroos, Macropus rufus, and western grey kangaroos, M. fuliginosus. Wildlife Research 18, 377–401.
| Density distributions and habitat associations of red kangaroos, Macropus rufus, and western grey kangaroos, M. fuliginosus.Crossref | GoogleScholarGoogle Scholar |
Calef, GW, DeBock, EA, and Lortie, GM (1976). The reaction of barren-ground caribou to aircraft. Arctic 29, 201–212.
| The reaction of barren-ground caribou to aircraft.Crossref | GoogleScholarGoogle Scholar |
Cañadas, A, Desportes, G, and Borchers, D (2004). The estimation of the detection function and g(0) for short-beaked common dolphins (Delphinus delphis), using double-platform data collected during the NASS-95 Faroese survey. Journal of Cetacean Research and Management 6, 191–198.
Catling PC, Coops NC (2004) Recent applications of airborne videography to management of forest fauna. In ‘Conservation of Australia’s forest fauna’. (Ed. D Lunney) pp. 537–548. (Royal Zoological Society of New South Wales)
Caughley, G (1974). Bias in aerial survey. Journal of Wildlife Management 38, 921–933.
Caughley, G, and Grice, D (1982). A correction factor for counting emus from the air, and its application to counts in Western Australia. Australian Wildlife Research 9, 253–259.
| A correction factor for counting emus from the air, and its application to counts in Western Australia.Crossref | GoogleScholarGoogle Scholar |
Caughley, G, Sinclair, R, and Scott-Kemmis, D (1976). Experiments in aerial survey. The Journal of Wildlife Management 40, 290–300.
| Experiments in aerial survey.Crossref | GoogleScholarGoogle Scholar |
Choquenot, D (1995). Assessing visibility bias associated with helicopter counts of feral pigs in Australia’s semi-arid rangelands. Wildlife Research 22, 569–578.
| Assessing visibility bias associated with helicopter counts of feral pigs in Australia’s semi-arid rangelands.Crossref | GoogleScholarGoogle Scholar |
Clancy, TF, Pople, AR, and Gibson, LA (1997). Comparison of helicopter line transects with walked line transects for estimating densities of kangaroos. Wildlife Research 24, 397–409.
| Comparison of helicopter line transects with walked line transects for estimating densities of kangaroos.Crossref | GoogleScholarGoogle Scholar |
Côté, SD (1996). Mountain goat responses to helicopter disturbance. Wildlife Society Bulletin 24, 681–685.
Cox, TE, Matthews, R, Halverson, G, and Morris, S (2021). Hot stuff in the bushes: thermal imagers and the detection of burrows in vegetated sites. Ecology and Evolution 11, 6406–6414.
| Hot stuff in the bushes: thermal imagers and the detection of burrows in vegetated sites.Crossref | GoogleScholarGoogle Scholar |
DeYoung, CA, Guthery, FS, Beasom, SL, Coughlin, SP, and Heffelfinger, JR (1989). Improving estimates of white-tailed deer abundance from helicopter surveys. Wildlife Society Bulletin 17, 275–279.
Elphick, CS (2008). How you count counts: the importance of methods research in applied ecology. Journal of Applied Ecology 45, 1313–1320.
| How you count counts: the importance of methods research in applied ecology.Crossref | GoogleScholarGoogle Scholar |
Elton CS (1927) ‘Animal ecology.’ (Sidgwick & Jackson: London, UK)
Evans M, Hastings N, Peacock B (1993) ‘Statistical Distributions.’ 2nd edn. (John Wiley & Sons: New York)
Fewster, RM, Laake, JL, and Buckland, ST (2005). Line transect sampling in small and large regions. Biometrics 61, 856–859.
| Line transect sampling in small and large regions.Crossref | GoogleScholarGoogle Scholar |
Fewster, RM, Southwell, C, Borchers, DL, Buckland, ST, and Pople, AR (2008). The influence of animal mobility on the assumption of uniform distances in aerial line-transect surveys. Wildlife Research 35, 275–288.
| The influence of animal mobility on the assumption of uniform distances in aerial line-transect surveys.Crossref | GoogleScholarGoogle Scholar |
Fleming, PJS, and Tracey, JP (2008). Some human, aircraft and animal factors affecting aerial surveys: how to enumerate animals from the air. Wildlife Research 35, 258–267.
| Some human, aircraft and animal factors affecting aerial surveys: how to enumerate animals from the air.Crossref | GoogleScholarGoogle Scholar |
Fleming, PJS, Choquenot, D, and Mason, RJ (2000). Aerial baiting of feral pigs (Sus scrofa) for the control of exotic disease in the semi-arid rangelands of New South Wales. Wildlife Research 27, 531–537.
| Aerial baiting of feral pigs (Sus scrofa) for the control of exotic disease in the semi-arid rangelands of New South Wales.Crossref | GoogleScholarGoogle Scholar |
Fryxell JM, Sinclair ARE, Caughley G (2014) ‘Wildlife ecology, conservation, and management.’ 3rd edn. (Wiley-Blackwell: Chichester, UK)
Glennie, R, Buckland, ST, and Thomas, L (2015). The effect of animal movement on line transect estimates of abundance. PLoS ONE 10, e0121333.
| The effect of animal movement on line transect estimates of abundance.Crossref | GoogleScholarGoogle Scholar |
Greene, K, Bell, D, Kioko, J, and Kiffner, C (2017). Performance of ground-based and aerial survey methods for monitoring wildlife assemblages in a conservation area of northern Tanzania. European Journal of Wildlife Research 63, 77.
| Performance of ground-based and aerial survey methods for monitoring wildlife assemblages in a conservation area of northern Tanzania.Crossref | GoogleScholarGoogle Scholar |
Grigg, GC, Beard, LA, Alexander, P, Pople, AR, and Cairns, SC (1999). Aerial survey of kangaroos in South Australia 1978-1998: a brief report focusing on methodology. Australian Zoologist 31, 292–300.
| Aerial survey of kangaroos in South Australia 1978-1998: a brief report focusing on methodology.Crossref | GoogleScholarGoogle Scholar |
Harrington, FH, and Veitch, AM (1991). Short-term impacts of low-level jet fighter training on caribou in Labrador. Arctic 44, 318–327.
| Short-term impacts of low-level jet fighter training on caribou in Labrador.Crossref | GoogleScholarGoogle Scholar |
Havens, KJ, and Sharp, EJ (1998). Using thermal imagery in the aerial survey of animals. Wildlife Society Bulletin 26, 17–23.
Hone, J (1986). Accuracy of the multiple regression method for estimating population density in strip transects. Australian Wildlife Research 13, 121–126.
| Accuracy of the multiple regression method for estimating population density in strip transects.Crossref | GoogleScholarGoogle Scholar |
Jachmann, H (2002). Comparison of aerial counts with ground counts for large African herbivores. Journal of Applied Ecology 39, 841–852.
| Comparison of aerial counts with ground counts for large African herbivores.Crossref | GoogleScholarGoogle Scholar |
Jarman, PJ (1974). The social organisation of antelope in relation to their ecology. Behaviour 48, 215–267.
| The social organisation of antelope in relation to their ecology.Crossref | GoogleScholarGoogle Scholar |
Jarman PJ (1991) Social behavior and organization in the Macropodoidea. In ‘Advances in the study of behavior. Vol. 20’. (Eds PJB Slater, JS Rosenblatt, C Beer, M Milinski) pp. 1–50. (Academic Press) https://doi.org/10.1016/S0065-3454(08)60318-6
Krausman, PR, Leopold, BD, and Scarborough, DL (1986). Desert mule deer responses to aircraft. Wildlife Society Bulletin 14, 68–70.
Laake, J, Dawson, MJ, and Hone, J (2008). Visibility bias in aerial survey: mark-recapture, line-transect or both? Wildlife Research 35, 299–309.
| Visibility bias in aerial survey: mark-recapture, line-transect or both?Crossref | GoogleScholarGoogle Scholar |
Lethbridge, M, Stead, M, and Wells, C (2019). Estimating kangaroo density by aerial survey: a comparison of thermal cameras with human observers. Wildlife Research 46, 639–648.
Lhoest, S, Linchant, J, Quevauvillers, S, Vermeulen, C, and Lejeune, P (2015). How many hippos (Homhip): algorithm for automatic counts of animals with infra-red thermal imagery from UAV. International Archives of the Photogrammetry, Remote Sensing and Spatial Information Sciences XL-3/W3, 355–362.
| How many hippos (Homhip): algorithm for automatic counts of animals with infra-red thermal imagery from UAV.Crossref | GoogleScholarGoogle Scholar |
Linklater, WL, and Cameron, EZ (2002). Escape behaviour of feral horses during a helicopter count. Wildlife Research 29, 221–224.
| Escape behaviour of feral horses during a helicopter count.Crossref | GoogleScholarGoogle Scholar |
Lunney, D, Purcell, B, McLeod, S, Grigg, G, Pople, T, and Wolter, S (2018). Four decades of research and monitoring the populations of kangaroos in New South Wales: one of the best long-term datasets in Australia. Australian Zoologist 39, 784–800.
| Four decades of research and monitoring the populations of kangaroos in New South Wales: one of the best long-term datasets in Australia.Crossref | GoogleScholarGoogle Scholar |
Marshall L (2019) Dssim: distance sampling simulations. R package version 1.1.4. Available at https://rdocumentation.org/packages/DSsim/versions/1.1.4
Melville, GJ, Tracey, JP, Fleming, PJS, and Lukins, BS (2008). Aerial surveys of multiple species: critical assumptions and sources of bias in distance and mark–recapture estimators. Wildlife Research 35, 310–348.
| Aerial surveys of multiple species: critical assumptions and sources of bias in distance and mark–recapture estimators.Crossref | GoogleScholarGoogle Scholar |
Packard, JM, Summers, RC, and Barnes, LB (1985). Variation of visibility bias during aerial surveys of manatees. The Journal of Wildlife Management 49, 347–351.
| Variation of visibility bias during aerial surveys of manatees.Crossref | GoogleScholarGoogle Scholar |
Palka, DL, and Hammond, PS (2001). Accounting for responsive movement in line transect estimates of abundance. Canadian Journal of Fisheries and Aquatic Sciences 58, 777–787.
| Accounting for responsive movement in line transect estimates of abundance.Crossref | GoogleScholarGoogle Scholar |
Pople, AR, Cairns, SC, Clancy, TF, Grigg, GC, Beard, LA, and Southwell, CJ (1998a). An assessment of the accuracy of kangaroo surveys using fixed-wing aircraft. Wildlife Research 25, 315–326.
| An assessment of the accuracy of kangaroo surveys using fixed-wing aircraft.Crossref | GoogleScholarGoogle Scholar |
Pople, AR, Clancy, TF, Thompson, JA, and Boyd-Law, S (1998b). Aerial survey methodology and the cost of control for feral goats in western Queensland. Wildlife Research 25, 393–407.
| Aerial survey methodology and the cost of control for feral goats in western Queensland.Crossref | GoogleScholarGoogle Scholar |
Pople, AR, Cairns, SC, Menke, N, and Payne, N (2006). Estimating the abundance of eastern grey kangaroos (Macropus giganteus) in south-eastern New South Wales, Australia. Wildlife Research 33, 93–102.
| Estimating the abundance of eastern grey kangaroos (Macropus giganteus) in south-eastern New South Wales, Australia.Crossref | GoogleScholarGoogle Scholar |
Reilly, BK, van Hensbergen, HJ, Eiselen, RJ, and Fleming, PJS (2017). Statistical power of replicated helicopter surveys in southern African conservation areas. African Journal of Ecology 55, 198–210.
| Statistical power of replicated helicopter surveys in southern African conservation areas.Crossref | GoogleScholarGoogle Scholar |
Rice, WR, and Harder, JD (1977). Application of multiple aerial sampling to a mark–recapture census of white-tailed deer. The Journal of Wildlife Management 41, 197–206.
| Application of multiple aerial sampling to a mark–recapture census of white-tailed deer.Crossref | GoogleScholarGoogle Scholar |
Ries, EH, Hiby, LR, and Reijnders, PJH (1998). Maximum likelihood population size estimation of harbour seals in the Dutch Wadden Sea based on a mark–recapture experiment. Journal of Applied Ecology 35, 332–339.
| Maximum likelihood population size estimation of harbour seals in the Dutch Wadden Sea based on a mark–recapture experiment.Crossref | GoogleScholarGoogle Scholar |
Samuel, MD, and Pollock, KH (1981). Correction of visibility bias in aerial surveys where animals occur in groups. The Journal of Wildlife Management 45, 993–997.
| Correction of visibility bias in aerial surveys where animals occur in groups.Crossref | GoogleScholarGoogle Scholar |
Schroeder, NM, and Panebianco, A (2021). Sociability strongly affects the behavioural responses of wild guanacos to drones. Scientific Reports 11, 20901.
| Sociability strongly affects the behavioural responses of wild guanacos to drones.Crossref | GoogleScholarGoogle Scholar |
Seber GAF (1982) ‘The estimation of animal abundance and related parameters.’ 2nd edn. (MacMillan: New York, NY, USA)
Short, J, and Hone, J (1988). Calibrating aerial surveys of kangaroos by comparison with drive counts. Australian Wildlife Research 15, 277–284.
| Calibrating aerial surveys of kangaroos by comparison with drive counts.Crossref | GoogleScholarGoogle Scholar |
Southwell, C (1994). Evaluation of walked line transect counts for estimating macropod density. The Journal of Wildlife Management 58, 348–356.
| Evaluation of walked line transect counts for estimating macropod density.Crossref | GoogleScholarGoogle Scholar |
Stankowich, T (2008). Ungulate flight responses to human disturbance: a review and meta-analysis. Biological Conservation 141, 2159–2173.
| Ungulate flight responses to human disturbance: a review and meta-analysis.Crossref | GoogleScholarGoogle Scholar |
Takada, H, Nakamura, K, and Minami, M (2019). Effects of the physical and social environment on flight response and habitat use in a solitary ungulate, the Japanese serow (Capricornis crispus). Behavioural Processes 158, 228–233.
| Effects of the physical and social environment on flight response and habitat use in a solitary ungulate, the Japanese serow (Capricornis crispus).Crossref | GoogleScholarGoogle Scholar |
Terletzky, PA, and Koons, DN (2016). Estimating ungulate abundance while accounting for multiple sources of observation error. Wildlife Society Bulletin 40, 525–536.
| Estimating ungulate abundance while accounting for multiple sources of observation error.Crossref | GoogleScholarGoogle Scholar |
Tracey, JP, and Fleming, PJS (2007). Behavioural responses of feral goats (Capra hircus) to helicopters. Applied Animal Behaviour Science 108, 114–128.
| Behavioural responses of feral goats (Capra hircus) to helicopters.Crossref | GoogleScholarGoogle Scholar |
Tracey, JP, Fleming, PJS, and Melville, GJ (2005). Does variable probability of detection compromise the use of indices in aerial surveys of medium-sized mammals? Wildlife Research 32, 245–252.
| Does variable probability of detection compromise the use of indices in aerial surveys of medium-sized mammals?Crossref | GoogleScholarGoogle Scholar |
Tracey, JP, Fleming, PJS, and Melville, GJ (2008). Accuracy of some aerial survey estimators: contrasts with known numbers. Wildlife Research 35, 377–384.
| Accuracy of some aerial survey estimators: contrasts with known numbers.Crossref | GoogleScholarGoogle Scholar |
van Hensbergen, HJ, Berry, MPS, and Jurits, J (1996). Helicopter-based line-transect estimates of some southern African game populations. South African Journal of Wildlife Research 26, 81–87.
Walter, MJ, and Hone, J (2003). A comparison of 3 aerial survey techniques to estimate wild horse abundance in the Australian Alps. Wildlife Society Bulletin 31, 1138–1149.
Witt, RR, Beranek, CT, Howell, LG, Ryan, SA, Clulow, J, Jordan, NR, Denholm, B, and Roff, A (2020). Real-time drone derived thermal imagery outperforms traditional survey methods for an arboreal forest mammal. PLoS ONE 15, e0242204.
| Real-time drone derived thermal imagery outperforms traditional survey methods for an arboreal forest mammal.Crossref | GoogleScholarGoogle Scholar |


